A Stupendous Assessment of Uncatalysed Bromate
Oscillator System Highlighting the Thermal Effect On Oxidative Bromination of P-Sulphonic Phenol by Acidic Bromate

Dr.Archana Garg

A Stupendous Assessment of Uncatalysed Bromate Oscillator System Highlighting the Thermal Effect On Oxidative Bromination of P-Sulphonic Phenol by Acidic Bromate

Dr.Archana Garg¹
Department of Chemistry, Maharshi Dayanand University, Haryana, India

Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

Abstract

p-Sulphonic phenol-Sulphuric acid-Bromate system is a one the most renowned uncatalysed Bromate Oscillator (UBO). This system is extremely sensitive to an increase in temperature and is manifested as changes in its various parameters. It was observed during present work that the temperature increase also changes the various parameters viz. induction period, Number of oscillations, time of last oscillation, time for colourisation of reaction mixture. Interestingly it was also noted that the period of oscillations has shown a considerable decrease.

Keywords

Uncatalysed bromate Oscillator (UBO), p-Sulphonic phenol, thermal, energy of activation, colour change

INTRODUCTION

Chemical oscillating reactions have attracted the attention of researchers for many years because of their challenging chemistry and their wealth of dynamic behaviour. 1-4Belousov-Zhabotinsky reaction is one of the most studied chemical oscillatory reactions which uses aliphatic (malonic acid, citric acid etc.) or aromatic compounds ( polyphenols and aniline derivatives). The BZ reaction with aliphatic substrate needs a catalyst like cerium, ferroin, etc, while BZ reaction with aromatic substrates commonly known as UBOs do not need a catalyst as the role of catalyst is taken over by the quinone/semiquinone produced in the reaction mixture during oxidation5. But presence of BZ catalyst has a pronounced effect on the uncatalysed system. Temperature has a marked effect on the UBOs 6, 7. We studied the influence of temperature on the p-Sulphonic phenol-Bromate-Sulphuric acid system without catalyst8. Experiments were carried out to see the effect of temperature on this system at the optimum concentration conditions i.e. at [p-sulphonic phenol] = 0.020 M , [H2SO4] = 1.44 M , [BrO3 -] = 0.032 M 8. The results show that in general the induction period (t in), number of oscillations, amplitude of oscillations, total duration of oscillation, period of oscillation (tp) vary significantly on changing the temperature. In p-Sulphonic phenol system raising the temperature from 303 K caused the induction period, number of oscillations and oscillation period decrease whereas there is no significant effect on amplitude of oscillations. The details of these effects are as follows:

EFFECT OF TEMPERATURE ON INDUCTION PERIOD

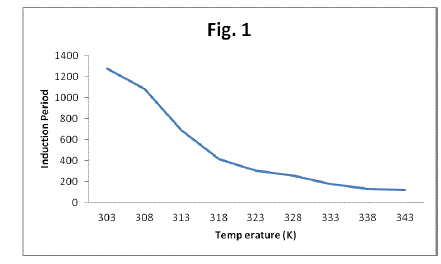
The results show that the induction period (t in) depend greatly on temperature. Raising the temperature made the induction period decrease (Table 1, Fig 1). For the appearance of first oscillation a critical concentration of bromo derivatives is to be accumulated in the reaction mixture. It is the rate of bromination which increases with the increase in temperature and the critical concentration of bromo derivatives is achieved at an early time thereby decreasing the induction period. Such a decrease in the induction period implies the involvement of the bromo derivative as an intermediate in the overall reaction7, 10-12.

Table 1 Effect of Temperature on Induction Period at [p-sulphonic phenol] = 0.020 M , [H2SO4] = 1.44 M ,

EFFECT OF TEMPERATURE ON TOTAL NUMBER OF OSCILLATIONS AND TOTAL DURATION OF OSCILLATIONS

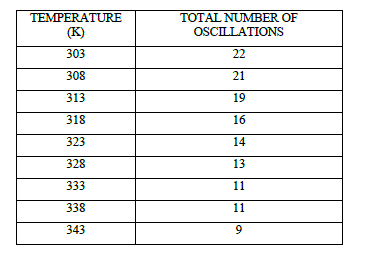
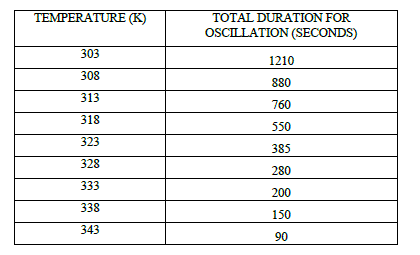
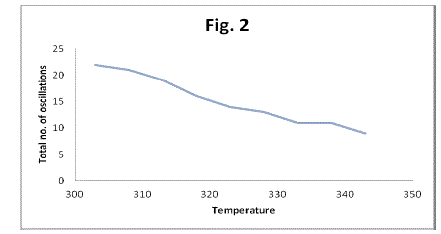
The number of oscillations and total duration of oscillations to occur decrease with the augmentation in the temperature from 303 K to 343 K (Table 2, 3, Fig 2, 3). In this system number of oscillations decreases with the increase of temperature and at 70 o c more or less chaotic oscillations were recorded. At higher temperature the system being closed, is driven more quickly to thermodynamic equilibrium; hence the decrease in the number of oscillations can be attributed to the fast consumption of substrate.

Table 2 Effect of Temperature on Total number of Oscillations at [p-sulphonic phenol] = 0.020 M , [H2SO4] = 1.44

Table 3 Effect of Temperature on Total time for Oscillations at [p-sulphonic phenol] = 0.020 M , [H2SO4] =

EFFECT OF TEMPERATURE ON COLOUR OF SOLUTION

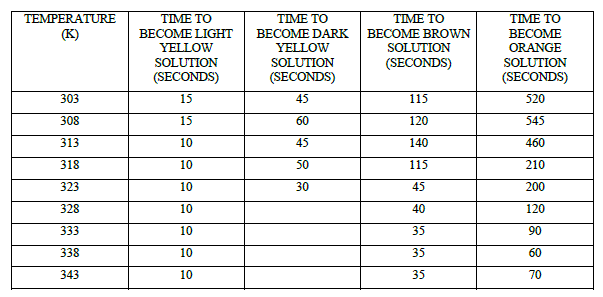
Bromate imparts colour to the system by forming bromo derivatives of the substrate which subsequently releases bromide ions on oxidation of bromo derivatives. It is evident from a gradual colour change as reported. (Table 4, Fig 4, 5, 6). It is interesting to observe that Bromate solution is the last constituent added to the reaction mixture and bromination of substrate start very quickly. Major portion of substrate gets brominated before the commencement of first oscillation5, 6. Progressively the colour of the reaction mixture becomes orange from light yellow, dark yellow, light brown and dark brown. In the present system longest time taken by the system to become orange is at 308 K. while temperatures above and below this temperature it takes lesser time. From the mechanistic point of view, the FKN mechanism seems to be satisfactorily consistent with our system 13,14.

Table 4 Effect of Temperature on Colour of the solution at [p-sulphonic phenol] = 0.020 M , [H2SO4] = 1.44 M , [BrO3 -] = 0.032 M

CALCULATION OF ENERGY OF ACTIVATION

apparent energy of activation is calculated from the slope of the graph between 1/T vs log 1/t where ‘T’ is the temp on absolute scale and ‘t’ is the Induction period , time for a fixed number of oscillations (nine oscillations) and total time of oscillations. (Table 5, 6, 7, Fig 7,8,9)

Table 5 Calculation of Apparent Energy of Activation (Induction Period) at [p-sulphonic phenol] = 0.020 M , [H2SO4]

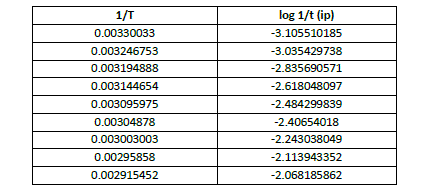Table 6 Calculation of Apparent Energy of Activation (for first nine oscillations) at [p-sulphonic phenol] = 0.020 M ,

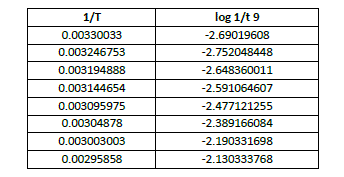Table 7 Calculation of Apparent Energy of Activation (for all oscillations) at [p-sulphonic phenol] = 0.020 M , [H2SO4] = 1.44 M , [BrO3 -] = 0.032 M

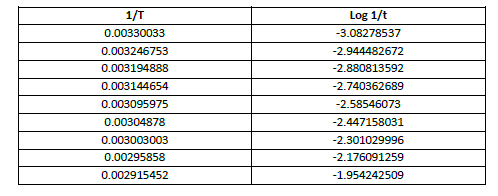The apparent activation energy Ea(induction period) was calculated from slope of the curve and is found to be 55.287 kJ mol-1, and that of oscillation period for total oscillations Ea(Total oscillations) was 54.705 kJ mol-1 , while the apparent energy of activation for first 9 oscillations was found to be 35.303k J mol-1. This data shows that the apparent energy of activation for induction period is slightly higher than the overall apparent energy of activation, although they could explain the difficulty degree of overall elementary reactions, Ea (induction) is comparable to Ea (Total oscillations) , they could illustrate the induction reaction is no more easier than that oscillation reaction which could be due to the presence of –sulphonic acid group at the para position. However the Ea value for first 9 oscillations is quite less.

References

Geest, T., Olsen, L.F., Steinmets, C.G., Larter, R., Shafer, W.M., ‘Nonlinear Analyses Of Periodic And Chaotic Time Series From The Peroxidase-Oxidase Reaction’, J. Phys. Chem., Vol. 97, pp.8431, 1993.
Strizhak, P.E., Didenko, O.Z., Ivashchenko, T.S., ‘Determination of Traces of Thallium using the Transient Chaotic Regime in the Belousov– Zhabotinskii Oscillating Chemical Reaction’, Anal. Chim. Acta, Vol. 428, Issue 1, pp.15-21, 2001.
Sasaki, Y., ‘Numerical Study on the Chaotic Response of the Minimal Chemical Oscillator to a Sinusoidal Perturbation’, Bull.Chem.Soc.Jpn., Vol. 72, No. 12 pp.2607-2615. 1999.
Yatsimirskii, K.B., Strizhak, P.E., Ivaschenko. S., ‘Potential of chaotic chemical systems in nanotrace analysis based on the Belousov- Zhabotinskii reaction (bro(-)(3)-malonic acid-ferroin). Determination of manganese(II)’, Talanta, , Vol. 40, No. 8, pp.1227-32, 1993.
Ramaswamy, R., Venkatachalam, S., and Sethuraman, S., ‘A New Double Substrate Uncatalyzed Bromate oscillator react.Kinet.Catal.Lett. Vol. 74, No. 1, pp. 41-49, 200.
Masood, A. N., Nadeem B. G., Rastogi, R. P., Peerzada, G. M., ‘Effect of Temperature on Oscillatory Behaviour of the System Containing Isomers of Hydroxybenzoic Acid in Batch Reactor’, E-Journal of Chemistry, Vol. 5, No. 4, pp.832-837, 2008.
Gull, U., Peerzada G. M., Ganaie, N. B., Dhar, N. A., ‘Effect of Initial Substrate Concentrations and Temperature on the Oscillatory Behavior of Phloroglucinol-Based Belousov–Zhabotinsky Reaction’ Bull. Chem. Soc. Jpn., Vol. 86, No. 2, pp. 266–272, 2013.
Garg, A., ‘Insights into Chemical Oscillatory Reactions through an Illustrious Case Study of p-Sulphonic Phenol: Sulphuric Acid: Bromate system research’, Journal of Pharmaceutical, Biological and chemical sciences, Vol. 4, No. 2, pp. 1738-1743, 2013.
Zuzana, L., Adamckova, P., Sevck, F., ‘Dynamic behavior during the oxidation of phenol with bromate in a closed reactor’, New J. Chem., Vol. 25, pp. 487-490, 2001.
Tockstein, A., Handlrova, M., ‘Study of the mechanism of the oscillating reaction between bromate and phenol’, Collect . Czech. Chem. Commun., Vol. 47, No. 9, pp. 2454-65, 1982.
Orban, M., Szokan G., ‘Intermediates and Products Formed During the Periodic Reaction Between Phenol and Bromate’, ACH, Models in Chemistry, Vol. 132, No. 1-2, pp.179-189,1995.
Ganaie, N. B., Peerzada, G. M.,‘Effect of Initial Reagent Concentrations on the Oscillatory Behaviour of the BZ Reaction in a Batch Reactor’, International Journal of Chemical Kinetics, Vol. 41, Issue. 10, pp.650–657, 2009.
Field, R. J., Koros, E., Noyes, R. M., ‘Oscillations in Chemical Systems: Thorough Analysis of Temporal Oscillation in the Bromate-Cerium- Malonic Acid System’ J. Am. Chem. Soc., Vol. 94, No. 25, pp.8649-8664, 1972.
Field, R. J., Noyes, R. M., ‘Oscillations in Chemical Systems: Limit Cycle Behaviour in a Model of a Real Chemical Reaction’, J. Chem. Phys., Vol. 60, No. 5, pp.1877-84, 1974.