International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

A Suzuki Type Unique Common Coupled Fixed Point Theorem in Metric Spaces

K.P.R.Rao1, K.R.K.Rao2, V.C.C.Raju3
  1. Professor, Dept. of Mathematics, Acharya Nagarjuna University, Nagarjuna Nagar-522 510, A.P., India
  2. Assistant Professor, Dept. of Mathematics, VBIT, Ghatkesar-501 301, A.P., India
  3. Professor, Department of Mathematics, University of Botswana, Private Bag UB 00704, Gaborone, Botswana
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

In this article, we study a unique common coupled fixed point theorem of Suzuki type for Jungck type mappings in metric spaces. Our result generalize and modify several comparable results in the literature.

Keywords
Coupled fixed point, metric space, weakly compatible maps.

Mathematics Subject Classification
54H25, 47H10.

INTRODUCTION AND PRELIMINARIES
Banach contraction principle plays a very important role in nonlinear analysis and has many generalizations.Recently Suzuki [ 31 ] proved generalized versions of both Banach’s and Edelstein’s basic results and thus initiated a lot of work in this direction, for example refer [3,4,5,8,13,18-21,24-28,31,32] and the references in them .
In 2006, Bhaskar and Lakshmikantham [29]introduced the notion of a coupled fixed point in partially ordered metric spaces,also discussed some problems of the uniqueness of a coupled fixed point and applied their results to the problems of the existence and uniqueness of a solution for the periodic boundary value problems. Later several authors obtained coupled fixed point theorems in various spaces,for example refer [1,2,6,7,9-12,14-17,22,23,29,30,33-36] and the references in them.
The aim of this paper is to combine the ideas of coupled fixed points and Suzuki type fixed point theorems to obtain a unique common coupled fixed point theorem for Jungck type mappings in a metric space.
First we give the following theorem of Suzuki [31].
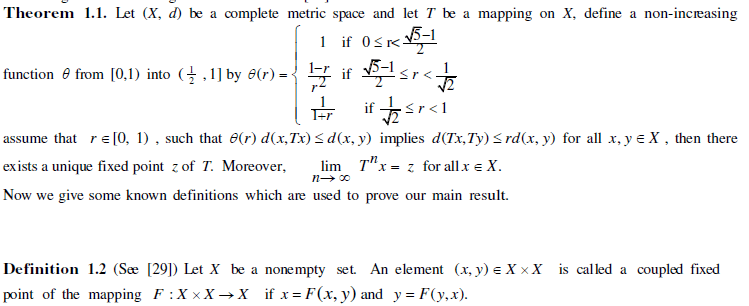
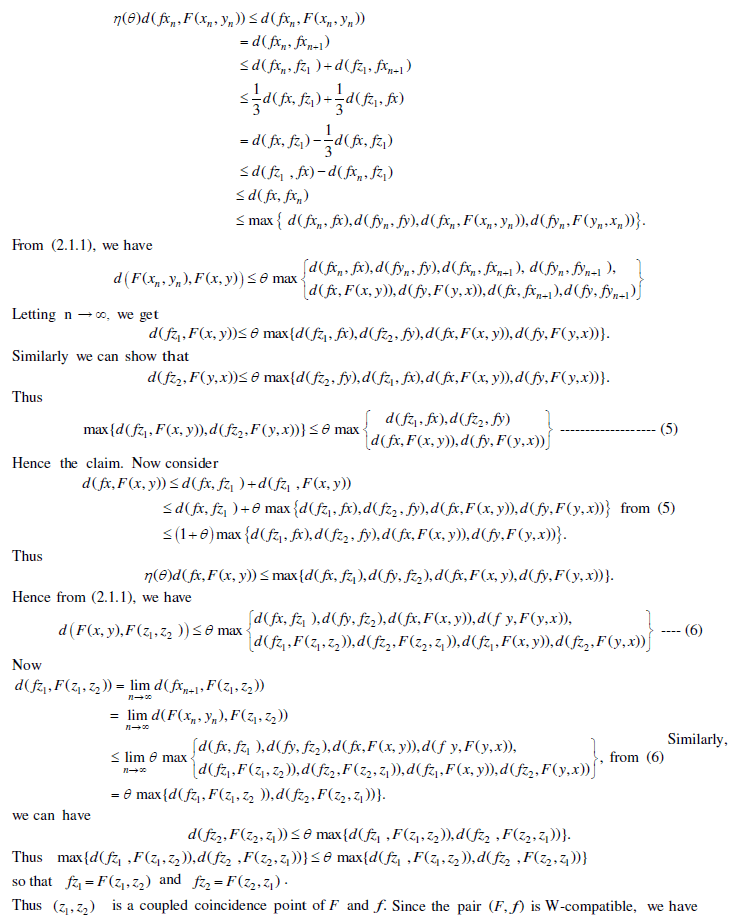
image
image
image
image
image

References
  1. B. Samet, “ Coupled fixed point theorems for a generalized Meir-Keeler Contraction in partially ordered metric spaces”, Nonlinear Anal., vol.72, 2010, pp. 4508-4517.
  2. B.S. Choudhury, A. Kundu, “A coupled coincidence point result in partially ordered metric spaces for compatible
  3. mappings”, Nonlinear Anal., vol. 73,no.8, 2010, pp.2524-2531.
  4. D.Doric Rade, Lazovic,Some “ Suzuki-type fixed point theorems for generalized multivalued mappings and applications”, Fixed point theory and Applications, vol. 2011, 2011 , 1/40, 8 pages.
  5. D.Doric, Z.Kadelburg, S.Radenovic, “Edelstein-Suzuki type fixed point results in metric and abstract metric spaces”, Nonlinear Analysis TMA, vol. 75, 2012, pp. 1927-1932.
  6. D.Paesano, Pasquale Vetro, “Suzuki’s type characterizations of com- pleteness for partial metric spaces and fixed points for partially ordered metric spaces”, Topology and its Applications, vol. 159, 2012, pp. 911-920.
  7. E. Karapinar, “Couple fixed point theorems for nonlinear contractions in cone metric spaces”, Comp. Math. Anal., vol. 59, 2010, pp. 3656 -3668.
  8. E. Karapinar, “Couple fixed point on cone metric spaces”, Gazi Univ. J.Sci., vol. 24, no. 1, 2011, p p . 51-58.
  9. E.Karapinar, “Edelstein type fixed point theorems”, Ann. Funct. Anal., vol. 2, no. 1, 2011, pp.51-58.
  10. F. Sabetghadam, H.P. Masiha, A.H. Sanatpour, “ Some coupled fixed point theorems in cone metric spaces”, Fixed Point Theory Appl., vol .2009, 2009, Article ID 125426, 8 pages.
  11. H. Aydi, “Some coupled fixed point results on partial metric spaces”. International Journal of Mathematics and Mathematical Sciences, vol. 2011, 2011, Article ID 647091, 11 pages.
  12. H. Aydi, E. Karapinar, W. Shatnawi, “ Coupled fixed point results for (φ − ψ) - weakly contractive condition in ordered partial metric spaces”, Comput. Math. Appl., vol. 62 no. 12 2011, pp. 4449 – 4460.
  13. H.S. Ding , L. Li, “ Coupled fixed point theorems in partial ordered cone metric spaces”, Filomat, vol . 25, no. 2, 2011, pp. 137-149.
  14. I.Altun , Ali Erduran, “ A Suzuki type fixed point theorem”, Internat. Math. Math. Sci., vol. 2011, Article ID 736063, 9 pages.
  15. K.P.R.Rao, S.Hima Bindu, Md.Mustaq Ali, “Coupled fixed point theorems in d-Complete topological spaces”, J.Nonlinear Sci.Appl. , vol. 5, 2012, pp. 186 -194.
  16. K.P.R.Rao , K.R.K.Rao , Erdal Karapinar , “ Common coupled fixed point theorems in d-complete topological spaces”, Ann. Funct. Anal., vol.3, no.2, 2012, pp. 107-114
  17. K.P.R.Rao, G.N.V.Kishore, V.C.C.Raju, “ A coupled fixed point theorem for two pairs of W-compatible maps using altering distance function in partial metric spaces,” Journal of Advanced Research in Pure Mathematics , vol .4, Issue 4, 2012, pp. 96- 114.
  18. M. Abbas, M. Ali Khan, S. Radenovic, “ Common coupled fixed point theorems in cone metric spaces for w-compatible mappings”, Appl. Math. Comput., vol . 217, 2010, p p . 195-202.
  19. M.Aggarwal, Renu Chugh, Raj Kamal, “Suzuki type fixed point results in G-metric spaces and Applications”, International Journal of Computer Applications, vol. 47, no. 12 , 2012, pp. 14-17.
  20. M.Kikkawa , T.Suzuki, “ Some similarity between contractions and Kan nan mappings, Fixed Point Theory Appl., vol. 2008 , 2008, 8 pages.
  21. M.Kikkawa, T.Suzuki, “Three fixed point theorems for generalized contractions with constants in complete metric spaces, Nonlinear Analysis , vol.69, 2008, pp. 2942-2949.
  22. N.Hussain, D.Doric, Z.Kadelburg , S.Radenovic, “Suzuki type fixed point results in metric type spaces, Fixed point theory and Applications, vol. 2012, 2012:126, 15 pages.
  23. N.V. Luong, N.X. Thuan, “Coupled fixed point theorems in partially ordered metric spaces”, Bull. Math. Anal.
  24. Appl., v o l . 2 , no. 4 , 2010, p p . 16-24.
  25. N. V. Luong , N. X. Thuan, “Coupled fixed points in partially ordered metric spaces and application”, Nonlinear Analysis. Theory, Methods and Applications, vol. 74, no. 3, 2011, 983 -992.
  26. Popescu O, Two fixed point theorems for generalized contractions with constants in complete metric spaces”, Cent. Eur. J. Math., vol. 7 , 2009, pp., 529-538 .
  27. Renu Chugh, Raj Kamal , Madhu Aggarwal, “Properties P and Q for Suzuki type fixed point theorems in metric spaces”, International Journal of Computer Applications, vol. 50, no. 1, 2012, 44-48.
  28. R.K.Bose, M.K.Roy Chowdhury, “Fixed point theorems for some generalized contractive multi valued mappings and fuzzy mappings”, Math Vesnik, vol. 63, no. 1, 2011, pp. 7-26.
  29. S.L.Singh, H.K.Pathak, S.N.Mishra, “ On a Suzuki type general fixed point theorem with applications”, Fixed point theory and Applications, vol.2010, Article ID 234717, 15 pages.
  30. S.L.Singh, S.N.Mishra, Renu Chugh, Raj Kamal, “General common fixed point theorems and applications”, Journal of Applied Mathematics, vol. 2012, 2012, Article ID 902312, 14 pages.
  31. T.G.Bhaskar , V.Lakshmikantham, “ Fixed point theorems in partially ordered cone metric spaces and applications”, Nonlinear Analysis: Theory,methods and Applications, 65(7)(2006),825-832.
  32. T. Gnana Bhaskar, V. Lakshmikantham, “ Fixed point theorems in partially ordered metric spaces and applications”,
  33. Nonlinear Analysis. Theory, Methods and Applications, vol. 65, no. 7, 2006, p p . 1379-1393.
  34. T. Suzuki, “A generalized Banach contraction principle that characterizes metric completeness”, Proceedings of the American Mathematical Society, vol. 136, no. 5, 2008 , pp. 1861-1869.
  35. T.Suzuki, “A new type of fixed point theorem in metric spaces”, NonlinearAnalysis TMA, vol. 71, 2009, pp. 5313 -5317.
  36. V. Lakshmikantham, L. C iric, “Coupled fixed point theorems for non- linear contractions in partially ordered metric spaces”, Nonlinear Analysis. Theory, Methods and Applications, vol. 70, no. 12, 2009, p p . 4341- 4349.
  37. W. Shatanawi, “Coupled fixed point theorems in generalized metric spaces, Hacettepe Journal of Mathematics and
  38. Statistics, vol. 40, no. 3, 2011, pp. 441- 447.
  39. W. Shatanawi, B. Samet, M. Abbas, “ Coupled fixed point theorems for mixed monotone mappings in ordered partial metric spaces”, Mathematical and Computer Modelling, vol. 55 , 2012, 680 - 687.
  40. W. Shatanawi, “On w-compatible mappings and common coupled coincidence point in cone metric spaces”, Applied Mathematics Letters, vol. .25, no. 6, 2012, pp. 925 - 931.