ISSN ONLINE(2278-8875) PRINT (2320-3765)
ISSN ONLINE(2278-8875) PRINT (2320-3765)
M. N. Rindani 1, A. A. Bavarva 2
|
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Advanced Research in Electrical, Electronics and Instrumentation Engineering
In next generation wireless communication and mobile systems‟ main requirement is to achieve higher data rates also with maximum bandwidth utilization. Now, for that MIMO communication method is used. In this paper, we represent EXTENDED ALAMOUTI scheme with 4X4 diversity order which is one of the diversity schemes used in order to improve the error performance. This paper discuses the error performance of this new diversity method on different fading channels mainly AWGN, RICIAN fading channel, RAYLEIGH fading channel and NAKAGAMI fading channel. This paper represents the error performance comparison of this new scheme and ALAMOUTI scheme with 2XM diversity order over all four fading channels with ML detector. This paper also represents the comparison among all four fading channel to elaborate the difference between them by using BER vs. SNR graph.
Keywords |
||||||||||||||
| ALAMOUTI, ML, AWGN, MIMO, BER. | ||||||||||||||
INTRODUCTION |
||||||||||||||
| Now days, MIMO technology has attracted more attention in case of wireless communication systems because of some efficient improvement in error performances, spectral efficiency, bandwidth utilization, higher throughput, etc.. Because of these improvements in wireless communication systems and cellular systems, it is used in many of the well known cellular technologies such as LTE (Long Term Evolution), 3GPP-LTE, CDMA-2000, Mobile Wi-MAX, WIBRO (Wireless Broadband Internet) (IEEE 802.16), WLAN (IEEE 802.11x) and some of the video broadcasting techniques such as DVB, DAB, DVB-T, etc.. [2-3]. | ||||||||||||||
| Now, in order to remove the effect of fading some of the interleaved coding schemes are used such as space-block codes based on transmit, receiver and transmit-receiver diversity schemes for MISO, SIMO and MIMO systems, respectively. One of the well known receiver diversity scheme is MRRC (Maximal Receiver Ratio Combining) scheme of the diversity order 1XM. Then in order to remove its disadvantages and in order to improve its performance two new schemes were developed by SIVASH M. ALAMOUTI which was then become one of the well known space time diversity scheme known as ALAMOUTI scheme as shown in [2]. | ||||||||||||||
| This paper organizes as follow: - Section-II includes the basic information about different fading channels and information about the channel parameter. The main part of the paper will start from Section-III which includes the theoretical concept of previously developed diversity schemes as in [2] and then new extension in that scheme with double code rate is shown. Then, next Section-IV includes the simulation results for all four fading channels and its comparison individually with each other and also with previous scheme for each fading channels for M-PSK modulation scheme. | ||||||||||||||
FADING & MIMO CHANNEL MODELS |
||||||||||||||
| MIMO wireless communication channel is the major and critical topic in wireless communication system. Main reason behind it is that wireless communication channel is more dynamic as compared to wired communication channel. Now, if we want to represent any MIMO communication channel, than we need to represent it in matrix form as shown in below equation – (1). Now, first of all consider the MIMO channel example shown in figure – (1):- | ||||||||||||||
 (1) (1) |
||||||||||||||
| Now, in above equation (1), each of the matrix elements hi,j , shows the impedance parameter of the channel between i -transmitting antenna and j - receiving antenna. So, the value of this hi,j parameter changes according to the value of LOS (Line-Of-Sight) parameter and fading distribution and fading effect according to the different channels. Now, before going towards the different types of fading channels, definition of fading is necessary. In most of the mobile or cellular systems, the height of the mobile antenna may be smaller than the surrounding structures. Thus, the existence of a direct or line-of-sight path between the transmitter and the receiver is highly unlikely. In such a case, propagation is mainly due to reflection and scattering from the buildings and by diffraction over and/or around them. So, in practice, the transmitted signal arrives at the receiver via several paths with different time delays creating a multi-path situation [5]. | ||||||||||||||
| At the receiver, these multi-path waves with randomly distributed amplitudes and phases combine to give a resultant signal that fluctuates in time and space. Therefore, a receiver at one location may have a signal that is much different from the signal at another location, only a short distance away, because of the change in the phase relationship among the incoming radio waves. This causes significant fluctuations in the signal amplitude. This phenomenon of random fluctuations in the received signal level is termed as fading [4-5]. Now, based on the fading distribution, variety of fading models is there, which are well-known in wireless communication system as a wireless communication channel. Now, here we are considering mainly four models: - | ||||||||||||||
| AWGN, RAYLEIGH, RACIAN and NAKAGAMI fade channel models:- | ||||||||||||||
| A. AWGN Channel | ||||||||||||||
| Additive white Gaussian noise (AWGN) channel is a universal channel model for analysing any new scheme. In this model, the channel does nothing but add a white Gaussian noise to the signal passing through it. Fading does not exist or if exists than it is of very less amount. The only distortion is introduced by the AWGN. AWGN channel is a theoretical channel used for analysis purpose only. | ||||||||||||||
| So, if s(t) is transmitted symbol then the received symbol is given as:- | ||||||||||||||
| Where η(t) is the Additive White Gaussian Noise (AWGN). | ||||||||||||||
| B. Rayleigh Channel | ||||||||||||||
| Rayleigh fading is statistical channel model for the representation of the effect of multi-path propagation environment over transmission symbols or radio signals. Now, when the transmitted signal passed through the channel will vary randomly or fade according to the Rayleigh distribution then that channel termed as RAYLEIGH channel. Rayleigh fading is mostly applicable only when there is no Line-Of-Sight between transmitter and receiver. | ||||||||||||||
| So, if s(t) is transmitted symbol then the received symbol is given as:- | ||||||||||||||
| Here R(t) is distributed by using Rayleigh distribution function as shown in [5]. | ||||||||||||||
| Where η(t) is the Additive White Gaussian Noise (AWGN). | ||||||||||||||
| C. Rician Channel | ||||||||||||||
| Rician fading channel is statistical model for radio propagation model for multipath propagation caused by partial affection of transmitted symbols itself. It means that Rician fading occurs when one of the paths, typically a line of sight signal is much stronger than others. So, the amplitude gain is characterized by a Rician distribution. So, if s(t) is transmitted symbol then the received symbol is given as:- | ||||||||||||||
| Here, received signal is distributed by Rician probability distribution function as in [5]. | ||||||||||||||
| D. Nakagami Channel | ||||||||||||||
| It is possible to describe both Rayleigh and Rician fading channel with change in only one parameter which is generally denoted mainly as „mâÃâ¬ÃŸ. Here, as we increase or decrease the value of the nakagami factor „mâÃâ¬ÃŸ, it changes the value of the probability distribution function towards the Rician or Rayleigh fading distribution, respectively. | ||||||||||||||
| As we choose the value of m<1 than Nakagami channel acts as a Rayleigh channel. Similarly, as we increase the value of nakagami factor i.e. when it is m>1 (m=1.5, 2, 4, etc.) than Nakagami channel acts as a Rician channel. So, we can see the different BER performances as we change the value of the nakagami factor m. [5] | ||||||||||||||
THEORETICAL BACKGROUND OF DIVERSITY SCHEME |
||||||||||||||
| This section will include the basic background of the ALAMOUTI scheme with 2xM diversity order as in [1-2] and the EXTENDED ALAMOUTI SCHEME with 4x4 diversity order. | ||||||||||||||
| A. Alamouti scheme | ||||||||||||||
| ALAMOUTI scheme for 2 transmitting antennas is illustrated in figure – (2). Now, here all the transmitting symbols those are two 1 s and 2 s are generally coded as shown in figure and then these coded symbols are generally interleaved in particularly two time-slots with the code rate of 2 symbols per second. It means two symbols 1 s and 2 s is transmitted during first time slot and another two coded symbols such as * 2 -s and * 1 s is transmitted during second time slot. | ||||||||||||||
| Now, these symbols are transmitted through the channel whose parameters are according to the no of transmitting and receiving antennas respectively as shown in equation-(1). Now, the receiving sequences are given by:- | ||||||||||||||
| Now, these received sequences are then given to the Alamouti decoder which consists of combiner and channel estimator. Output of the combiner is given as below equation-(6):- | ||||||||||||||
| Where, | ||||||||||||||
 (7) (7) |
||||||||||||||
| So, the output of the combiner, which is then given to the ML detector in order to detect the symbols and then they are compared with the transmitted symbols s1 and s2 in order to develop the error performance for ALAMOUTI 2x2 diversity technique. | ||||||||||||||
| B. Extended Alamouti scheme | ||||||||||||||
| Now, for better error performance this ALAMOUTI 2xM scheme is generally extended for 4 transmitting antennas as shown in [7] for MISO case that is for 4x1. Now, it is extended for 4x4 in order to achieve better error performance as well as code rates. | ||||||||||||||
| Now, the extended Alamouti 4x4 scheme is illustrated in figure (3). Now, here all the transmitting symbols those are four s1, s2, s3 and s4 are coded and interleaved within four time-slots in such a way that it provides the code-rate of 4 symbols per time-slots. This coded transmission symbol matrix and other required circuitry is shown in block diagram format in below figure-(3):- | ||||||||||||||
| Now, these symbols are transmitted through the channel whose parameters are according to the no of transmitting and receiving antennas respectively as shown in equation-(1). Now, the receiving sequences are given by: - | ||||||||||||||
| Now, these received sequences are then given to the Alamouti decoder which consists of combiner and channel estimator. Output of the combiner is given as below equation-(6):- | ||||||||||||||
| So, the output of the combiner, which is then given to the ML detector in order to detect the symbols and then they are compared with the transmitted symbols s1, s2, s3 and s4 in order to develop the error performance for this diversity technique. | ||||||||||||||
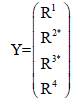 (10) (10) |
||||||||||||||
| Where:- | ||||||||||||||
| & another factor is given by equation – (11) which is shown below: - | ||||||||||||||
 (11) (11) |
||||||||||||||
SIMULATION RESULTS & DISCUSSION |
||||||||||||||
| Now, this section is the main part that is results of the BER vs. SNR for representing the error performances of different diversity schemes described in previous section. Now, this section includes the simulation results for M-PSK (QPSK) modulation schemes and for four fading channels. These results are developed using MATLAB software. | ||||||||||||||
| Now, firstly the figure 4 to figure 7 represents the results for QPSK according to the different fading channels. As shown in the figure 4, it includes the results for Alamouti 2X1, Alamouti 2X2 and extended Alamouti 4X4 scheme for AWGN channel. Now as shown in figure 4 new extended scheme provides better error performance as compared to other schemes in terms of coding and diversity gain with higher code rate of 4 symbols per timeslots. | ||||||||||||||
| As shown in the figure 5, it includes the results for Alamouti 2X1, Alamouti 2X2 and extended Alamouti 4X4 scheme for Rician fading channel. Now as shown in figure 5 new extended scheme provides error performance similar & some amount of better as compared to 2X2 and 2X1, respectively. Here, we generally got the worst result in case of Rayleigh fading channel with worst case of fading parameters. But, still extended scheme provides the double code rate of 4 symbols per timeslots. | ||||||||||||||
| Here, below figure-7 shows the results of the error performance for the one of the well known fading channel i.e. nakagami fading channel. As the nakagami factor is increased from 0.5 to 4 then, the error performance results moving towards the Rician fading channel and as we decreases the nakagami factor value from 1 than, the error performance results moving towards the Rayleigh fading channel for all schemes. | ||||||||||||||
| Here, below table – I shows the comparison of all wireless channels for the different values of SNR for extended Alamouti scheme with 4x4 diversity order. | ||||||||||||||
CONCLUSION |
||||||||||||||
| From the simulation results and Table- I, it is concluded that for less faded (AWGN) and for heavily faded with Line-Of-Sight (RICIAN) channels ALAMOUTI 4x4 scheme provide better coding gain and diversity gain as compared to ALAMOUTI 2x2 scheme. But, for highly faded channel (RAYLEIGH) it provides the worst result as compared to 2x2 ALAMOUTI scheme. Similarly in NAKAGAMI fading channel as the value of nakagami factor „mâÃâ¬ÃŸ increased from 0.5 to 4, it provides the better results and error performance moves from Rayleigh to Rician channel, respectively. So, AWGN channel provides the better results compared to other channels because of the less fading. Then Rician channel provides better results as compared to Rayleigh channel. For higher order modulation scheme its error performance is degraded. | ||||||||||||||
| ACKNOWLEDGMENT | ||||||||||||||
| Authors wish to express their gratitude to MATLAB7.0 for the simulation results described in this paper. Authors also gratefully acknowledge the contributions of S. M. Alamouti, V. Tarokh, H. Jafarkhami, A.R. Calderbank and M.Rupp for their work on Space time block codes and diversity techniques and useful to the new extension of this paper. | ||||||||||||||
Tables at a glance |
||||||||||||||
|
||||||||||||||
Figures at a glance |
||||||||||||||
|
||||||||||||||
References |
||||||||||||||
|