International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Cone Metric Spaces and Common Fixed Point Theorems for Generalized Multivalued Mappings

S. K. Tiwari1, R .P .Dubey2, A. K. Dubey3
  1. Asst. Professor, Department of Mathematics, Dr. C.V. Raman University, Bilaspur, Chhattisgarh, India
  2. Professor, Department of Mathematics, Dr. C.V. Raman University, Bilaspur, Chhattisgarh, India
  3. Asst. Professor, Department of Mathematics , Bhilai Institute of Technology Bhilai house, Durg India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

Let P be a sub set of Banach space E and P is normal and regular cone on E; we generalize and obtain some sufficient conditions for the existence of common fixed point of multivalued mappings satisfying contractive type conditions in cone metric spaces. Our results unify, generalize and complement the comparable results from the current literature.

Keywords
Common fixed point, Cone metric spaces, multivalued mapping, Normal cone and non- normal cone

INTRODUCTION AND PRELIMINARIES
Fixed point theory plays a basic role in applications of various braches of mathematics, from elementary calculus and linear algebra to topology and analysis. Fixed point theory is not restricted to mathematics and this theory has many applications in other disciplines. This theory is closely related to game theory, military, economics, statistics and medicine. Much work has been done involving fixed points for multivalued contractions and none expansive maps using the Hausdorff metric was initiated by Markin [1].Later, an interesting and rich fixed point theory for such maps was developed. Nadler Jr. [2] has proved valtivalued version of the Banach contraction principle which states that each closed bounded valued contraction map on a complete metric space has a fixed point(see also[3],[4],[5],[6],[7]and[8]).
Quiet recently, Huang and Zhang [9] introduced the concept of cone metric space, replacing the set of positive real numbers by an ordered Banach space. He also gave the condition in the setting of cone metric space. These authors also studied the strong convergence to a fixed point with contractive constant in metric space and introduce the corresponding notion of completeness. Subsequently many authors have generalized the results of Huang and Zhang [9] and have studied fixed point theorems for normal and non-normal cone (see [10]). S. Hoon .Cho and Mi Sun Kim [11] have proved certain fixed point theorems using Multivalued mapping in the setting of contractive constant in metric spaces and also S. Hoon Cho and J.S. Base [12] proved fixed point theorems for multivalued mappings in cone metric spaces. R. C. Dimri, Amit Singh and Sandeep Bhatt[13] also proved common fixed point theorems for two multivalued maps in complete metric spaces with normal constant M=1. Further, Mujahid Abbas, B.E.Rhoades and Talat Nazir [14] obtained and generalized sufficient conditions for the existence of common fixed points of multivalued mapping satisfying contractive conditions in non-normal cone metric space.Wardowski [15] introduced the concept of multivalued contractions in cone metric spaces and, using the notion of normal cones, obtained fixed point theorems for such mappings.
The purpose of this paper is to prove some common fixed point results for multivalued mappings taking normal and non-normal in cone metric spaces. Our results extend and unify various comparable results in literature [16], [17], and [18]
Definition 1.1 [9] Let E be a real Branch space and P a subset of E. Then P is called a cone if it is satisfied the following conditions,
(I) P is closed, non-empty and P ≠ {0};
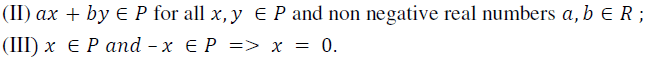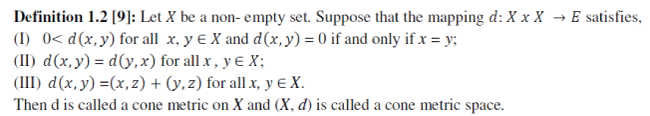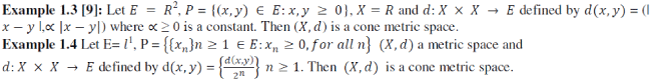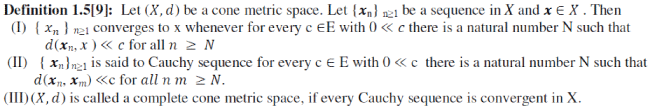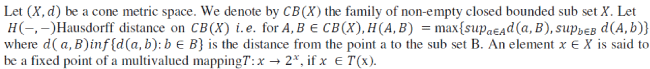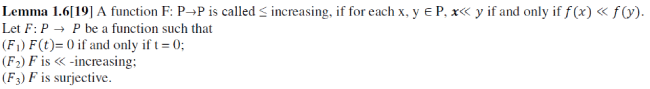image
image
image
image
image
image
image
image
image
image
image
image
image

References
  1. Markin, J.T. “Continuous dependence of fixed point set”, Proc. Amer. Math. Soc. 38,545-547, 1973.
  2. Nadler, jr. S. B., “Multivalued contraction mapping,” Pacific J. Math., 30 475-488, 1969.
  3. Ciric L. B. “A generalization of Banach contraction Principle”, Proc. Amer. Math Soc., 263- 273, 1974.
  4. Fisher, B. “A fixed point theorems for compact metric space,” Pub. Inst. Math. 25,193- 194, 1976.
  5. Jaggi, D. S.“Some unique fixed point theorems,” I. J. P. Appl. 8, 223-230, 1977.
  6. N air, S. V. R. , “Fixed point theorems”, Pure and Applied Mathematika Sic. 12, 73-77,1995
  7. Rani, D. and Chug, R. “Some fixed point theorems on contractive type mapping,” Pure and Applied Mathematika Sic. 41, 153-157, 1990
  8. Yadava, R. N., Rajput S. S. and Bhardwaj, R.K., “Some fixed point theorems for Extension of Banach contraction principle”, Acta Ciencia India 33, (2), 461-466, 2006.
  9. Huang Gaung, Zhang Xian. “Cone metric spaces and fixed point theorems of contractive Mappings”, J. MATH. Anal. Appl. 332, 1468-1476, 2007.
  10. Rezapour, Sh., Hamlbarani, R. “some notes on the paper- Cone metric spaces and fixed Point theorems of contractive mappings”, J. Mathe, Anal. Appl. 345, 719-724, 2008.
  11. Cho, Seong-Hoon, and Kim Mi Sun, “ Fixed point theorems for general contractiv Multi-valued mappings”, J. Appl. Math. Informatics, 27,343-350, 2009
  12. Cho, Seong- Hoon, and Base , J. S. “Fixed point theorems for multivalued maps in cone Metric spaces”, Fixed point theory Appl., 87 , 2011.
  13. Dimri, R.C. Amit singh, and R.C. Bhatt, R.C. “Common fixed point theorems for Multi-valued maps in cone metric spaces, ” Int. Math. Forum 5, 2271-2278, 2010.
  14. Abbas, Mujahid B.E. Rhoades and Talat Nazir, “Common fixed points of generalized Contractive multivalued mappings in cone metric spaces”, Math. Commune, 14, 365-378, 2009.
  15. Wardowski, D. “End points and fixed point of set-valued contractions in cone metric Spaces”, Nonlinear Analysis: Theory, Methods Applications, 71,512-516, 2009.
  16. Jain, Saurabh and Badshah .H. “Fixed point theorem of multivalued mappings in cone Metric spaces”, Int .J. Math. Archive -2, 2753-2756, 2011.
  17. R.Krishhnakumar, M. Marudai, Fixed point theorems of Multivalued Mappings in Cone Metric Spaces, Int. J. Contempt, 5,(2010), 1533-1540.
  18. Piyush Bhatnagar, Abha Tenguria, R. N. Yadava, and Rajesh Shrivastava, Some fixed and common fixed point theorems in metric spaces, Res. J. Of Pure Alg.2,(2012) 82-87.
  19. Seong-Hoon Cho, Fixed point theorems for generalized contractive mappings on cone metric spaces, Int. Journal of Math. Anal, 6 (2012), 2473-2481.