International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Efficient Domination number and Chromatic number of a Fuzzy Graph

Dr.S.Vimala1 J.S.Sathya2
  1. Assistant Professor,Department of Mathematics,Mother Teresa Women’s University, Kodaikanal, India
  2. Research Scholar, Department of Mathematics, Mother Teresa Women’s University, Kodaikanal, India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

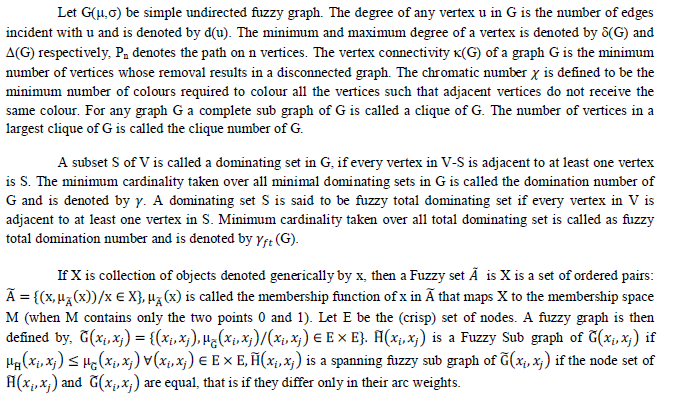
A subset S of V is called a domination set in G if every vertex in V-S is adjacent to at least one vertex in S. A dominating set is said to be Fuzzy Total Dominating set if every vertex in V is adjacent to at least one vertex in S. Minimum cardinality taken over all total dominating set is called as fuzzy total domination number and is denoted by 􀟛􀯙􀯧 (G). The minimum number of colours required to colour all the vertices such that adjacent vertices do not receive the same colour is the chromatic number 􀟯(G). For any graph G a complete sub graph of G is called a clique of G. In this paper we find an upper bound for the sum of the fuzzy total domination and chromatic number in fuzzy graphs and characterize the corresponding extremal fuzzy graphs.

Keywords
Fuzzy Efficient Domination Number, Chromatic Number, Clique, Fuzzy Graphs

INTRODUCTION
image
The fuzzy definition of fuzzy graphs was proposed by Kaufmann [4], from the fuzzy relations introduced by Zadeh [9]. Although Rosenfeld [5] introduced another elaborated definition, including fuzzy vertex and fuzzy edges. Several fuzzy analogs of graph theoretic concepts such as paths, cycles connectedness etc. The concept of domination in fuzzy graphs was investigated by A.Somasundram, S.Somasundram [6]. A. Somasundram presented the concepts of independent domination, total domination, connected domination and domination in cartesian product and composition of fuzzy graphs([7][8]).
image
image
image
image

III.CONCLUSION
In this paper, upper bound of the sum of Efficient domination and chromatic number is proved. In future this result can be extended to various domination parameters. The structure of the graphs had been given in this paper can be used in models and networks. The authors have obtained similar results with large cases of graphs for which image

References
  1. Teresa W. Haynes, Stephen T. Hedemiemi and Peter J. Slater “Fundamentals of Domination in graphs”, Marcel Dekker, Newyork 1998.
  2. Hanary F and Teresa W. Haynes , “Double Domination in graphs”, ARC Combinatoria 55, pp. 201-213. 2000
  3. Haynes, Teresa W., “Paired domination in Graphs”, Congr. Number 150, 2001
  4. Mahadevan G, Selvam A, “On independent domination number and chromatic number of a graph”, Acta Ciencia Indica, preprint 2008
  5. Paulraj Joseph J. and Arumugam S. “Domination and connectivity in graphs”, International Journal of Management and systems, 8 No.3:
    233-236. 1992
  6. Kaufmann.A., “Introduction to the theory of Fuzzy Subsets”, Academic Press, Newyork. 1975
  7. Rosenfield,A., Fuzzy graphs In: Zadeh, L.A., Fu, K.S., Shimura, M.(Eds), “Fuzzy sets and their applications” (Academic Press, New York)
  8. Somasundaram.A, Somasundaram,S, “Domination in Fuzzy Graphs – I”, Pattern Recognition Letters, 19, pp-787-791.,1998
  9. Somasundaram.A, , “Domination in Fuzzy Graphs – II”, Journal of Fuzzy Mathematics, 20. 2004
  10. Somasundaram.A, “Domination in Product of Fuzzy Graphs”, International Journal of Uncertainity , Fuzziness and Knowledge-Based
    Syatems, 13(2), pp.195-205. 2005
  11. Zadeh,L.A. “Similarity Relations and Fuzzy Ordering”, Information sciences, 3(2),pp.177-200. 1971
  12. Mahadevan G, “On domination theory and related concepts in graphs”, Ph.D. thesis, Manonmaniam Sundaranar University,Tirunelveli,India. 2005
  13. Paulraj Joseph J. and Arumugam S. “Domination and colouring in graphs”. International Journal of Management and Systems, Vol.8
    No.1, 37-44. 1997
  14. Paulraj Joseph J, Mahadevan G, Selvam A. “On Complementary Perfect domination number of a graph”, Acta Ciencia India, vol.
    XXXIM, No.2,847, 2006.
  15. Vimala S, Sathya J.S, “Graphs whose sum of Chromatic number and Total domination equals to 2n-5 for any n>4”, Proceedings of the
    Heber International Conference on Applications of Mathematics and statistics, Tiruchirappalli pp 375-381, 2012