ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| Dr. S. R. Pathak1, A. N. Dalvi2 Professor, Dept of Civil Engg., College of Engineering, Pune, Maharashtra, India1 Research Scholar, Dept of Civil Engg., College of Engineering, Pune, Maharashtra, India2 |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
Liquefaction can be defined as a loss of strength and stiffness in soils.The damage caused by seismic soil liquefaction is generally diverse and extensive. Real time identification of liquefied sites is thus the foremost step in the study of soil liquefaction engineering, which can be achieved by analysing surface ground motion parameters and dynamic response of soil. The prediction of dynamic responses requires knowledge of dynamic soil properties whereas ground motion is usually characterised with the help of amplitude, frequency and duration parameters. The authors of this paper intend to investigate the role of dynamic soil properties in Liquefaction Potential (LP) assessment. This technical note thus presents an elementary approach to evaluate LP based on dynamic response of soils.
Keywords |
||
| Liquefaction Potential (LP), Dynamic soil properties, Empirical Liquefaction Model, ground motion parameters | ||
INTRODUCTION |
||
| The nature and distribution of earthquake damage such as liquefaction is strongly influenced by the response of soils to cyclic loading. Dynamic response of soils to cyclic loading can be characterised in terms of dynamic properties of soils and the ground motion parameters. Evaluation of LP of a saturated sandy deposit during an earthquake requires knowledge of the intensity and duration of cyclic shear stresses of shaking as well as the cyclic shear resistance of the soil. Generally, cyclic shear stresses could be assessed through simplified procedures (Seed et al. 1983; Seed and Idriss 1971; Seed 1979; Seed 2010) or based upon results of a site response analysis. The cyclic shear resistance of soils could be evaluated in the laboratory or based upon empirical relationships using in-situ material parameters e.g., SPT, CPT, or Vs (Finn 2002; Seed et al.1985; Youd et al. 2001). Phatak and Pathak (1999) have already developed similar such model “model A” separating “yes “ and “ no” zones of liquefaction based on field performance data. Further, these authors have also invented a method to evaluate triggering acceleration indicating initiation of liquefaction. The triggering acceleration is defined based on the relation between LP and corrected SPT blow count (Pathak and Phatak 2005). | ||
| Authors of the present paper have worked on recently developed empirical liquefaction models and reaffirmed the fact that most of these models are data specific (Pathak and Dalvi 2011). Further, it is observed that the model based on one of the dynamic soil properties (Vs) performs better than the models based on other in-situ indices. Based on quantitative evaluation of performance of the empirical models it is believed that considering dynamic soil properties in LP evaluation procedures may improve their performance which can be used for a wide range of databases.Accordingly, a preliminary approach to evaluate LP in terms of dynamic soil properties and other ground motion parameters is discussed as follows. | ||
II. LIQUEFACTION POTENTIAL ASSESSMENT |
||
| The most widely used method for evaluating liquefaction is the stress-based procedure first proposed by Seed and Idriss, 1971 given by; | ||
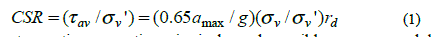 |
||
| where, CSR = cyclic stress ratio representing seismic demand on soil layer; amax = peak horizontal acceleration at the ground surface generated by the earthquake; g= acceleration due to gravity; sv and sv’ are total and effective vertical overburden stresses respectively and rd = stress reduction coefficient. It is noted that conventional methods consider only peak ground acceleration (amax) to reflect incident seismic motion and thus neglect the spectral characteristics of the input motion. However, the ratio of (vmax/amax) is an informative measure that can account for the frequency content of input motions. (Jafarian et al, 2010). Orense (2005) has shown that combination of vmax and amax is a good indicator of occurrence and non-occurrence of liquefaction during earthquakes. Similar approach to detect liquefaction occurrence using seismic records has been developed by various researchers such as, Trifunac (1995), Towahata et al. (1997), Kayen and Mitchell (1997). Further, while considering inclusion of appropriate dynamic soil properties in LP assessment, it is observed that for large earthquake magnitudes and long duration, small strain shear modulus, Gmax is a key parameter. Moreover, Gmax represents the soil stiffness which is influenced mainly by cyclic strain amplitude, density and mean effective stress of soil, and number of loading cycles. Thus the number of loading cycles of earthquake shaking is another important factor affecting soil behavior which can be related to the duration of strong ground motion. It is well established fact that an increase in overburden stresses, (s0’) increases susceptibility of soils to cyclic liquefaction hence plays major role in liquefaction assessment at a site. Based on above discussion, small strain shear modulus, (Gmax), frequency content in terms of the ratio vmax/amax, duration of strong ground motion(dur), and effective overburden pressure, (s0’) are selected as the most relevant parameters to evaluate LP more accurately. Hence, in accordance with the correlations of these parameters with liquefaction occurrence the functional form has been selected as; | ||
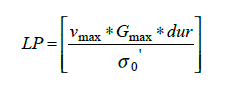 (2) (2) |
||
| where, ‘vmax’ is the horizontal strong motion velocity (m/sec), ‘Gmax’ is small strain shear modulus (kPa), ‘dur’ is duration of strong ground motion (sec), s0’ is initial effective overburden pressure (kPa). Further, to assess liquefaction occurrence at a site, it is required to compare the liquefaction potential with an in situ index that represents liquefaction resistance such as SPT, CPT or Vs. Using this principle as stated by eq. 2, Dalvi (2009) graphically showed that separation of liquefaction and non-liquefaction sites is possible as shown in Fig 1 below; | ||
| The dotted line in Fig 1 is purely meant for representaion purpose to indicate regions of liquefaction and non-liquefaction. The summary of parameters for earthquakes employed to represent the above separation is as given in Table.1. | ||
| Although the number of data points as seen in Fig. 1 is limited, it is worth mentioning that combination of dynamic soil properties and relevant ground motion parameters as stated by eq. 2 above bears the potential scope in LP assessment. Further research in this reagrd is in progress, an attempt is being made by authors of this article to develop an empirical liquefaction model based on methodology presented herein to represent the dynamic response more closely. | ||
III. CONCLUSION |
||
| An elementary approach to evalute LP in terms of small strain shear modulus, Gmax, peak ground velocity, vmax, duration of strong motion,dur and effective overburden pressure (s0 ’) is briefed. Application of proposed methodology to detect liquefaction occurrence is shown. The present approch appears to have potential application in assessment of LP. Further, it is anticipated that liquefaction occurrence can be more accurately detected using this criteria. In line with this, further investigation is being carried out to develop an efficient empirical liquefaction model which can be used for wide range of dataset to assess liquefaction susceptibility at an earthquake site. | ||
ACKNOWLEDGMENT |
||
| The authors would like to thank Department of Civil Engineering of College of Engineering Pune, An autonomous Institute of Government of Maharashtra for providing infrastructural support for the ongoing research work. | ||
Tables at a glance |
||
|
||
Figures at a glance |
||
|
||
References |
||
|