ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| Sajid Ali1, Rashid Ali2 Assistant Professor, Department of Mechanical Engineering, ACN, Aligarh, U.P, India1 Guest Faculty, Department of Mechanical Engineering, ZHCET, AMU, Aligarh, U.P, India 2 |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
The internal convection currents generated during the cooling process affect convective heat transfer coefficient from the surface of the container, these convection currents may increase the effective value of the surface film conductance (h). Therefore, in such situation the Nu-Re correlations, which are generally used to predict h-values, may not yield realistic results. In the present work, this effect has been investigated by using the empirical correlation developed through Temperature-Time measurements at the centre of liquid food containers during cooling .The main concern of the present work is about considering the heat transfer behaviour for liquid foods for which a cylindrical shape container of brass metal have taken, in this work the transient Time-Temperature relation is utilized to calculate the value of convection heat transfer coefficient (h) for each measured temperature at the centre of the cylinder (ro=0). Then after plotting the graph between „h‟ and „T‟ an expression between h and T is obtained, which is fed back in the programme developed with the help of finite difference method by which Time-Temperature variation is obtained. Experimental procedure was used to determine surface film conductance of cylindrical Apple and Orange juice container, calculated temperatures have been compared with the experimental results when the measured surface film conductance were used to solve the transient heat conduction equation in cylindrical coordinates. A consistently excellent agreement was observed.
Keywords |
||||||||||||||||
| Convection currents, Convective heat transfer coefficient, surface film conductance, liquid foods | ||||||||||||||||
INTRODUCTION |
||||||||||||||||
| Heat transfer analyses during cooling of liquid food containers are very complex phenomenon. Preservation of perishable commodities particularly food products either in solid or liquid form is one of the most common uses of mechanical refrigeration. As food products must be kept in a preserved condition during transient and subsequent storage until they are finally consumed. Many food items, particularly fruits and vegetables, that are hydrous solid bodies, are seasonal, since they are produced only during certain seasons of the year. They must be stored and preserved if they are to be made available throughout the year. The surface heat transfer coefficient can be determined experimentally by both steady and unsteady state methods. In the present work experiments were designed and fabricated. The apparatus consists of a refrigeration system using R-12 as refrigerant and cooling duct. Air was made to flow over cooling coils and it was cooled in the process. Cooled air was circulated through the duct. A cylindrical container filled with fruit juice sealed with rubber cork at both ends was hanged with the help of thread in the duct and blast of cooled air is allowed to pass over its outer surface. Thermocouple was inserted in the cylindrical container to note down the temperature of fruit juice for different time intervals and temperature was recorded by suitable arrangements at desired locations (i.e. at the centre) in the cylindrical container. Experimental procedure was used to determine surface film conductance of cylindrical Apple and Orange, juice container. Kopelman etâÃâ¬ÃŸ al [1] have used the classical method of Carslaw and Jaegar to solve the transient heat conduction equations for simple product geometry. Charan [2] has described a method which avoids the use of surface temperature and which determine the heat transfer coefficient from the time-temperatures measurements at the centre of an ice block, which was immersed in the freezing medium. Ramsey etâÃâ¬ÃŸ al [3] have used a conduction model to calculate the surface heat transfer coefficient of fresh cut, sweet potato flesh from experimental temperature measurements. The temperature variations were measured at an interior point in the potato and calculations were made to find the surface temperature, heat flux, and heat transfer coefficient. The empirical correlations reported by Ansari and his co-workers [4, 5, 6, 7] were found to predict effective values of surface film conductance for regular shaped bodies through transient time-temperature measurements. The reliability of the method was tested statically, and it was found to give satisfactory results. Dyer etâÃâ¬ÃŸ al [8] assumed the humidity transfer from the food product to the circulating air, which was considered as unsaturated. Their model yielded a cooling rate, which was faster than the measured cooling rate in the initial stages of cooling, and it becomes slower than the measured cooling rate during the later stages. On the other hand the analytical method developed by Badri Narayan [9] and Krishnamurthy and co-workers [10, 11, 12] used for unsaturated air-stream, yielded an overall faster cooling rate compared to the actual one. In the initial stages of cooling the difference between the measured and calculated was small but it becomes more pronounced during the later stages. | ||||||||||||||||
II MATHEMATICAL FORMULATION |
||||||||||||||||
| General form of the unsteady state or transient heat conduction in 3-D for non-homogenous (non-isotropic) and selfinternal heat generation material, in the absence of external force field and internal mass diffusion, may be written as [11]. | ||||||||||||||||
| Simplifying assumptions | ||||||||||||||||
| 1. The product is homogenous, | ||||||||||||||||
| 2. The internal heat generation is neglected and | ||||||||||||||||
| 3. The heat transfer is in radial direction. | ||||||||||||||||
| Combining above equations can be written in the following generalized form; | ||||||||||||||||
| Where, m=0 for slab, 1 for cylinder, 2 for the sphere r=distance of the point under consideration from the central axis of the cylinder or the centre of sphere. Initial and boundary condition during pre cooling The initial condition is given below by the equation | ||||||||||||||||
| Centre boundary condition | ||||||||||||||||
| Surface boundary condition If only convective heat transfer is considering at the product surface, the boundary condition will be defined by the equation | ||||||||||||||||
| If the effect of moisture content is also taken into consideration the surface boundary condition will be modified to include the latent heat of respiration and will be defined by the equation | ||||||||||||||||
| This completes the mathematical formulation for the transient heat transfer during precooling. Non dimensional heat transfer equation: In order to develop a generalized and versatile mathematical model it is necessary to non-dimensionalize the above equations. The dimensionless numbers used for this purpose are those that are most commonly used by food technologist and given as | ||||||||||||||||
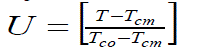 (8) (8) |
||||||||||||||||
 (11) (11) |
||||||||||||||||
| The cooling medium temperature (T cm) depends upon the actual physical situation of the process. Simultaneous heat and mass transfer: When energy is transferred from the product surface by convection as well as desiccation the minimum temperature that can be reached in this case is the wet bulb temperature of the cooling air so that T cm = T wb. the above facts are taken into account while the above dimensionless parameters are applied. Substitutions of the equation reduce the equation to the following equation respectively. | ||||||||||||||||
 (12) (12) |
||||||||||||||||
 (14) (14) |
||||||||||||||||
| The following correlations were developed and reported by Ansari etâÃâ¬ÃŸ al [5, 6] for cylindrical bodies: | ||||||||||||||||
 (16) (16) |
||||||||||||||||
| Where | ||||||||||||||||
| With known temperature-time history ro and k, the value of „hâÃâ¬ÃŸ may be calculated from equation (16). Nu-Re correlation for surface film conductance: Experimental time-temperature measurements by Ansari [13] for different fruits and vegetables was used to determine the thermal diffusivity using equation the required value of surface film conductance was calculated from the air stream velocity by the Nu-Re relationship available in the literature and is given in table I [6]. | ||||||||||||||||
III RESULTS AND DISCUSSIONS |
||||||||||||||||
| In present work, the variations of „hâÃâ¬ÃŸ with produced temperature have been considered. For each sample the „hâÃâ¬ÃŸ value, temperature data has been fitted and temperature dependent quadratic equation of „hâÃâ¬ÃŸ has been develops. These quadratic best fit plots of surface film conductance with temperature and their corresponding equations, as shown in figures 1, 2, 3 and 4 for each produce investigated. These equations were used to calculate variable „hâÃâ¬ÃŸ at each computational time-step using the temperature one time step earlier at the nodal points under consideration. Pure convective boundary condition was used with this effective „hâÃâ¬ÃŸ value and equation was solved to predict transient temperature other significant parameters, thermo-physical properties and measured temperature histories of all the orange, apple samples were chosen from the literature [14]. The computational temperature has been plotted against time as shown in figures 5, 6, 7and 8. These figures 5- 8 are also included measured temperature so the predicted and actual values may be compared. For establishing and validity and authenticity of the present scheme of calculations of temperature-time calculation were also made with the mathematical models available in the literature. The model with pure convective surface boundary condition defined earlier by the equation [7] was used. Other properties and parameters were the same as those used with present model. The plots of the time-temperature calculated by this scheme are also indicated in the figures 5 to 8 a look at these plots shows that the rate of cooling by this model consistently slower compared to its actual rate. This means a higher cooling time shall be predicted by mathematical model. It is observed from the temperature-time plots that the present scheme of calculation yields much improved results as compared to the other scheme available in the literature and commonly used. The time-temperature plots by the schemes of the present author results into a consistently remarkable agreement between the computed and experimentally measured temperatures. The agreement is particularly excellent at the later stages of cooling. Incidentally the later stages of cooling are improved from the point of view of calculation of precooling or processing time. | ||||||||||||||||
| In the given plots orange 1and orange 2 are for first sample of orange and second sample of orange juices, similarly in case of apple juice. | ||||||||||||||||
IV CONCLUSION |
||||||||||||||||
| In the present work, the surface film conductance is calculated from the transient temperature-time records at the centre of cylindrical containers of apple and orange juices. This was found to be very high in the beginning and at the later stages, it decreases with the lowering temperature. The variations are approximated by non linear equations. Temperature estimations are made first by assuming constant „hâÃâ¬ÃŸ value by Nu-Re relation. It is then repeated by taking variable „hâÃâ¬ÃŸ value calculated in the present work. The two estimated temperatures are plotted on the same graph. Temperature yielded by the variable „hâÃâ¬ÃŸ values are found to be consistently in much better agreement with measured values as compared to those yielded by constant „hâÃâ¬ÃŸ values. This established that the present approach of using variable „hâÃâ¬ÃŸ values for liquid foods is improved and more reliable. | ||||||||||||||||
| NOMENCLATURE | ||||||||||||||||
| A Area (m2) | ||||||||||||||||
| Bi Biot number (dimensionless) | ||||||||||||||||
| Cp Specific heat capacity ( KJ/Kg K) | ||||||||||||||||
| h Convective heat transfer coefficient (W/m2 K) | ||||||||||||||||
| k Thermal conductivity (W/m K) | ||||||||||||||||
| Km Mass transfer coefficient (Kg/m2 s) | ||||||||||||||||
| L Latent of heat of fusion on volume basis (J/m3) | ||||||||||||||||
| M Mass (Kg) | ||||||||||||||||
| Nu Nustle number (dimensionless) | ||||||||||||||||
| P Pressure (N/m2) | ||||||||||||||||
| Pr Prandtl number (dimensionless) | ||||||||||||||||
| Q Rate of heat flow (W) | ||||||||||||||||
| q Rate of heat flow per unit area (W/m2) | ||||||||||||||||
| Re Reynolds number (dimensionless) | ||||||||||||||||
| T Temperature (K) | ||||||||||||||||
| t Time (s) | ||||||||||||||||
| U dimensionless temperature | ||||||||||||||||
| V Volume (m3) | ||||||||||||||||
| W Specific humidity ratio | ||||||||||||||||
| w Water content (% by mass) | ||||||||||||||||
| R Non-dimensional space coordinate (=r/ro) | ||||||||||||||||
| r Space coordinate | ||||||||||||||||
| ro Characteristic radius(m) | ||||||||||||||||
| Greek Letters: | ||||||||||||||||
| α Thermal diffusivity (m2/s) | ||||||||||||||||
| θ Weighing factor for the implicit-explicit scheme | ||||||||||||||||
| φ Relative humidity (%) | ||||||||||||||||
| ρ Mass density (Kg/m3) | ||||||||||||||||
| τ Fourier number dimensionless (=αt/ro 2) | ||||||||||||||||
| Subscripts and Superscripts: | ||||||||||||||||
| a Air | ||||||||||||||||
| c Continuous phase | ||||||||||||||||
| cm Cooling medium | ||||||||||||||||
| d Dispersed phase | ||||||||||||||||
| db Dry bulb | ||||||||||||||||
| e Effective | ||||||||||||||||
| i Space point (in the space time mesh) | ||||||||||||||||
| in Initial | ||||||||||||||||
| j Time point (in the space time mesh) | ||||||||||||||||
| L Latent | ||||||||||||||||
| m Moist | ||||||||||||||||
| s Saturated | ||||||||||||||||
| se Sensible | ||||||||||||||||
| sf System final | ||||||||||||||||
| si System initial | ||||||||||||||||
| v Vapour | ||||||||||||||||
| t Total | ||||||||||||||||
Tables at a glance |
||||||||||||||||
|
||||||||||||||||
Figures at a glance |
||||||||||||||||
|
||||||||||||||||
References |
||||||||||||||||
|