International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Remarks on-multifunctions via filters

M. Lellis Thivagar , M.Anbuchelvi
  1. Professor and Head, School of Mathematics, Madurai Kamaraj University,Madurai,Tamil Nadu,India
  2. Associate Professor in Mathematics,V.V.Vanniaperumal College for Women,Virudhunagar,Tamilnadu,India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

Aim of this paper is to obtain a new class of separation known as ˆ -compact Spaces. Their properties are investigated in terms of nets, filterbase and ˆ -complete accumulation point. Also I -lower (resp.upper) ˆ -continuous and ˆ -multifunctions have been introduced to study ˆ -compact spaces.

Keywords
Ωˆ -closed sets., Ωˆ -complete accumulation point., Ωˆ -compactness., Ωˆ -multifunctions. 2010 Mathematics Subject Classification : 57C05, 57D05.

INTRODUCTION
Compactness is one of the most useful and fundamental notions of not only general topology but also for other advanced branches of Mathematics. Lellis Thivagar et.al [5] recently introduce the class of Ωˆ -closed sets which form a topology and properly lies between the class of δ -closed sets and that of ω -closed sets. The aim of this paper is to investigate some characterizations of Ωˆ -compact Spaces in terms of nets and filterbase. By introducing the notion of Ωˆ -complete accumulation points, we investigate some characterizations of Ωˆ -compact Spaces. This paper is to introduce concepts such as I -lower (resp.upper) Ωˆ -continuous and Ωˆ -multifunctions by which Ωˆ -compactness is studied. Also some characterizations of Ωˆ -multifunctions is obtained.

PRELIMINARIES
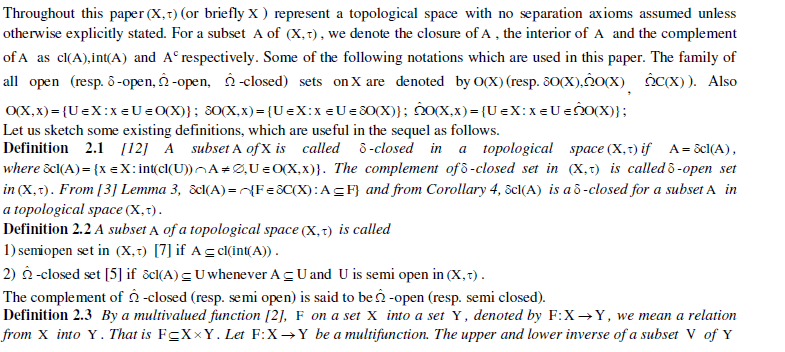image
image

Ωˆ -COMPACT SPACES VIA FILTERS
image
image
image
image
image
image
image
image
image

References
  1. AKDAG. M(Sivas),"On Super Continuous Multifunctions",Acta Math.Hungar.99 (1-2),(2003),143-153,
  2. Berge.C,"Topological Spaces",Macmillan,Newyork,1963.
  3. Ekici.E, "On  -semiopen sets and a generalizations of functions", Bol.soc.paran.Mat.(3s.),v.23,1-2,(2005:)73-84.
  4. Kovacevic.I,"Subsets and Paracompactness",Univ U Novom Sadu ,Zb Rad Prirod Mat. Fak Ser Mat.14,1984,79-87. [5] Lellis Thivagar M, Anbuchelvi M,"Note on ˆ sets in topological spaces" ,Mathematical Theory and Modeling,Vol 2,NO.9,2012,p.50-58.
  5. Lellis Thivagar M, Anbuchelvi M,"New spaces and Continuity via ˆ -closed sets",(Accepted)
  6. Levin.N, "Semi open stets and semi continuity in topological spaces",Amer.math.monthly ,70,1963,36-41.
  7. Neubrunn.T,"Strongly quasi continuous multivaluaed mappings in general topology and its relations to modern analysis and algebra VI (Prague 1986) Berlin,Heldermann,1988,p.351-59",
  8. Popa.V," Some properties of H-almost continuous ",problemy Mat, 10, 1988, 9-26
  9. Popa.V,Noiri.T," On upper and lower  -continuous multifunctions",R.Anal Exchange, 22, 1996/97, 362-76
  10. Popa.V,Noiri.T," On upper and lower  -continuous multifunctions",Math slovaca, 43, 1996, 88-96
  11. Velico.N.V,"H -closed topological spaces",Amer.math.soc.Trans, 78,1968, 103-18.
  12. Wine.D,"Locally Paracompact Spaces",Glasnik Mat. 10(30), 1975, 351-57.