Some Unique Common Fixed Point Theorems
In Parametric S-Metric Spaces

K.P.R.Rao; D.Vasu Babu; E.Taraka Ramudu

Some Unique Common Fixed Point Theorems In Parametric S-Metric Spaces

K.P.R.Rao¹ , D.Vasu Babu² , E.Taraka Ramudu³

Professor , Department of Mathematics, Acharya Nagarjuna University, Nagarjuna Nagar-522510, A.P.,India
Research Scholar, Department of Applied Mathematics, Krishna University - Dr.M.R.Appa Row Campus, Nuzvid-521 201,Krishna Dt.,A.P.,India
Asssistant professor, Department of Science and Humanities, Nova College of Engineering and Technology, Jupudi, Krishna Dt., A.P.,India

Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

Abstract

In this paper, we introduced parametric s-metric space and proved two unique common fixed point theorems in it. Our result generalizes and improves two main results of Ionescu,Rezapour and Samei [4].

Keywords

Parametric s-metric space, compatible maps, Weakly compatible maps.

2000 Mathematics Subject Classifications : 47H10, 54H25.

I. INTRODUCTION

The concept of fuzzy sets was intiated by Zadeh [10] in 1965 . The fuzzy metric space was introduced by Kramosil and Michalek [8]. Also, Grabeic[11] proved the contraction principle in the setting of fuzzymetric space. Also, George and Veeramani [1] modified the notion of fuzzy metric space with the help of continuous t-norm, by generalizing the concept of probablistic metric space to fuzzy situation. Later several authors, for example Vasuki [13],Pant[15] , Mishra et al.[14] ,Bari et al.[3], Vetro et al. [5] etc. proved fixed and common fixed point theorems in fuzzy metric spaces. We now state the following

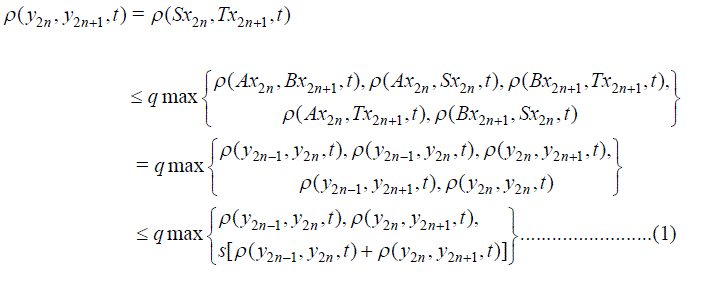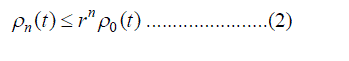Then (M, N) is called an intuitionistic fuzzy metric on X . The functions M(x, y,t) and N(x, y,t) denote the degree of nearness and the degree of non-earness between x and y with respect to t , respectively.

Definition 1.6[4]: Let (X,M,N,*,ÃÂ¯ÃÆÃÂ ) be an intuitionstic fuzzy metric space. The fuzzy metric (M, N) is called triangular whenever,

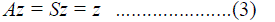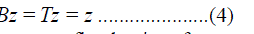References

A.George, P.Veeramani, on some results in fuzzy metric spaces, Fuzzy sets and systems,64 (1994), 395-399.
B.Schweizer, A.Sklar, statistical metric spaces,Pacific J.Math, 10(1960), 314-334.
C.Di Bari and C. Vetro, A fixed point theorem for a family of mappings in a fuzzy metric space, Rend. Ciric. Math.Palermo 52, (2003), 315-321.
C. Ionescu, Sh.Rezapour, M.E. Samei,Fixed pints of some new contractions on intuitionistic fuzzy metric spaces, Fixed point Theory and Appl., 2013( 2013):168.
C.Vetro,D.Gopal and M.Imdad, Common fixed point theorems fo ( ÃÂ¯ÃÂÃÂ¦ ,ÃÂ¯ÃÂÃÂ¹ )-weak contractions in fuzzy metric spaces, Indian J.Math,52(3),(2010),573-590.
G.Jungck,Compatible mappings and common fixed points,Int.J.Math.Math.Sci.,9(4),(1986),771-779.
G.Jungck and B.E.Rhoades,Fixed points for set-valued mappings without continuity,Indian J.Pure.Appl.Math.,29(3),(1998),227-238.
I.Kramosil and J. Michlek, Fuzzy metric and statistical metric spaces, Kybernetika, 11 (1975), 336-344.
J.H.Park,Intuitionstic fuzzy metric spaces,chaos solitons Fractals, 22(2004),1039-1046.
L.A.Zadeh, Fuzzy sets, Information and control,8(1965), 338-353.
M.Grabiec, Fixed points in fuzzy metric spaces, Fuzzy sets and systems, 27(1989), 385-389.
N.Hussain, S.Khalehiadeh, P.Salimi, Afra A.N.Abdou, A new approach to fixed point resultsin triangular intuitionstic fuzzy metric spaces, (A pre print).
R.Vasuki, A common fixed point theorem in a fuzzy metric space,Fuzzy sets and systems, 97(1998), 395-397.
S.N.Mishra,N.Sharma and S.L.Singh,Common fixed point of mappings on fuzzy metric spaces, Internat.J.Math.Math.Sci, 17(1994),253-258.
V.Pant, some fixed point theorems in fuzzy metric spaces, Tamkang J.Math. 1(2009),59-66.