ISSN ONLINE(2319-8753)PRINT(2347-6710)
ISSN ONLINE(2319-8753)PRINT(2347-6710)
| Avinash Kharat1, V. V. Kulkarni2 Research Scholar, Dept. of Production Engineering, K. I. T.?s College of Engineering, Kolhapur, Maharashtra, India1 Professor, Dept. of Production Engineering, K. I. T.?s College of Engineering, Kolhapur, Maharashtra, India.2 |
| Related article at Pubmed, Scholar Google |
Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology
This paper reviews some of the current developments in the determination of stress concentration factor in pressure vessels at openings. The literature has indicated a growing interest in the field of stress concentration analysis in the pressure vessels. The motivation for this research is to analyze the stress concentration occurring at the openings of the pressure vessels and the means to reduce the effect of the same. Most of the researchers have worked on the stress concentration occurring at circular and radial openings in the shell under internal pressure. Also some of the researchers have worked on holes in the end covers. In this paper the recent developments, theories for estimation of stress concentration are presented and the scope for future studies is also presented.
Keywords |
||||||
| stress distribution, stress concentration, stress concentration factor, pressure vessels | ||||||
INTRODUCTION |
||||||
| Pressure vessels find wide applications in thermal and nuclear power plants, process and chemical industries, in space and ocean depths, and fluid supply systems in industries. The failure of pressure vessel may result in loss of life, health hazards and damage of property. Due to practical requirements, pressure vessels are often equipped with openings of various shapes, sizes and positions. Vessels have openings to accommodate manholes, handholds, and nozzles. Openings vary in size from small drain nozzles to full vessel size openings with body flanges. The openings cannot be avoided because of various piping or measuring gauge attachments. They allow for the mounting of equipment, the insertion of instrumentation, and the connection of piping facilitating the introduction and extraction of content but they also lead to the high stress concentration which leads to the failure of pressure vessel. Openings in pressure vessels are frequent, in fact all riveted constructions make use of such means of fabrication, and all vessels must have openings. These geometric discontinuities alter the stress distribution in the neighborhood of discontinuity so that elementary stress equations no longer prevail. Such discontinuities are called „„stress raisers?? and the regions in which they occur are called the areas of stress concentrations. In this paper the contribution of various researchers in determination of stress concentration at opening in pressure is summarized for design of pressure vessel by using different approaches. The section III-A describes literature review related to openings in cylinder/shell. Where the section III-B describes literature review about openings in flat plate and section III-C describes the literature review about stress concentration factor at opening. All the literature is summarized in the form of table in each section. | ||||||
II. STRESS CONCENTRATION |
||||||
| In a cylindrical shell weakened by a hole, the stress distribution caused by an internal pressure load applied to the shell will differ considerably from that in an un-weakened shell. The maximum stress will be much larger if there is a circular hole in the shell than in the case where there is no penetration. This causes the rise in the stress distribution around the hole, to study the effect of stress concentration and magnitude of localized stresses, a dimensionless factor called Stress Concentration Factor (SCF), is used to calculate the stress rising around hole. The determination of S.C.F includes basic concept of engineering like maximum stress/strain and nominal stress etc. This factor is ratio between the maximum average stress generated in the critical zone of discontinuity and the stress produce over the cross section of that zone. Kt as defined by Eq. (1) is used. | ||||||
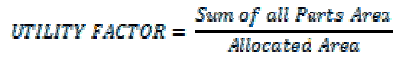 |
||||||
III. STRESS CONCENTRATION AT OPENINGS IN PRESSURE VESSELS – A REVIEW |
||||||
| For the study of stress concentration at opening at pressure vessel an extensive literature survey is carried out. | ||||||
| A. Literature review of openings on cylinder/shell: | ||||||
| Albert Kaufman (1965) correlated theoretical stresses and strains in a pressure vessel having a reinforced opening with experimental data obtained for progressive stages of elastic-plastic deformation up to the point of failure. Elastic-plastic stresses are used with the true ultimate tensile strength of the shell material to give a reasonably accurate prediction of the burst pressure. C. R. Calladine (1966) applied plastic design approach for the design of reinforcement for openings in thin spherical pressure vessels. The essence of the approach is to adjust the thickness and shape of the vessel in the vicinity of the opening so that the full limit pressure of the vessel may be carried with relatively little bending action. It is concluded that pad-reinforced nozzle represents major advantage over other forms of reinforcement is that its general form enables the forces on the nozzle and its surround due to pressure loading to be carried almost entirely by membrane stresses; and this in turn results in low stress concentration effects. R. Kitching (1970) compared the experimental limit pressures for cylindrical shells with unreinforced openings for different shapes and sizes. It was concluded that the limit pressures similar for openings of the same overall dimensions and is not dependent of the shape. M. N. Bapurao (1971) studied stresses around a small elliptic hole in an infinitely long circular cylindrical shell subjected to torsion. It was concluded that the effect of curvature for a given β is maximum if the hole is circular. As the hole is made more and more slender keeping β constant, the perturbation stress tend to decrease except for the crack case (λ = 1) when they become singular at the crack tip. A. J. Durelii (1973) presented work on the stresses concentration in a ribbed cylindrical shell with a reinforced circular hole subjected to internal pressure, by several experimental methods and the results obtained were compared with those corresponding to a non-reinforced hole in a ribbed and un-ribbed shell and also to a reinforced hole in an un-ribbed shell. From the result it was found that the maximum value of hoop stress, and longitudinal stress, in shells always occurred at the points θ = 0° and θ = 90°, respectively, along the edge of the hole, θ being the angle measured clockwise from the longitudinal axis of the hole R. C. Gwaltney (1973) compared theoretical and experimental stresses for spherical shells having single non-radial nozzles. The stress distributions for radial and non-radial nozzle geometry are analyzed. Stress distributions for the non-radial and the radial nozzle attachments are quite similar but the non-radial nozzle configuration gave the maximum normalized stress, both theoretical and experimental, for internal pressure and for axial loads on the nozzle as well as for pure bending moment loading in the plane of obliquity. M. S. Iyer (1975) presented work on analysis of a pressure vessel junction by the finite element method with different types of shell elements and concluded that quadrilateral shell element can be confidently applied for the analysis of pressure vessel problems. J. W. Bryson (1977) presented work on parametric study on stresses in reinforced nozzle-to-cylinder attachments under internal pressure loading as analyzed by the finite-element method. It was concluded from the study that both of the reinforcement designs investigated significantly reduce maximum stresses relative to configurations having little or no reinforcement. For internal pressure loading, neither of the reinforcement designs offered a significant advantage over the other in that both types of reinforcement gave very nearly the same maximum stresses. Dennis Martens (1996) presented a method which allows the vessel engineer to more accurately evaluate the flexibility and stresses in vessel nozzles within the time and expense parameters associated with the normal design process. It is concluded that it is desirable to use the Finite Element approach to better assess both the stiffnesses and the stresses in vessel nozzles. Kang Soo Kim (1999) presented work on opening distance patterns on the stress distribution of the pressure vessel head, using IDEA FEM code. Result indicated that the stress values of head parts between the adjacent nozzles increases according to the reduction of the distance between them. L.M.Bildy (2000) presented work for calculating the local primary stress from internal pressure in nozzle openings using beam on elastic foundation theory. M. Giglio (2000) compared two different methods for the construction of pressure vessel nozzles, designed with the same safety coefficient, according to ASME and VSR 1995 standards. It defines numerical and experimental analysis of behavior under low-cycle fatigue for pulsating pressure. In particular, a nozzle with integral reinforcement, designed according to ASME standards, is compared to a nozzle with external reinforcement (applied reinforcement plate) designed according to VSR 1995 standards with the same safety coefficient. J.S. Liu (2000) studied optimal shapes of intersecting pressure vessels using a novel topology/shape optimization method, called Metamorphic Development. Two types of intersecting structures, distinguished by flush and protruding nozzles, are investigated. The optimum problems of minimum mass intersecting structures are found by growing and degenerating simple initial structures subject to stress constraints. The optimization seeks to eliminate the stress peaks caused by the opening. It is shown that the design with a protruding nozzle would produce a better stress distribution than the design with a flush nozzle. You-Hong Liu (2004) presented work on Limit pressures and corresponding maximum local membrane stress concentration for two orthogonally intersecting thin-walled cylindrical shells subjected to internal pressure. The local membrane SCF at the intersections of two cylindrical shells subjected to the limit pressure load is calculated by elastic thin shell theoretical solutions. 3D finite element method (using ANSYS) in which the material is elastic-perfectly plastic is used. G. Rahimi (2005) presented the analyses in dimensionless form for a wide range of geometric parameters which are practically of interest. Analytical estimation of upper bound and lower bound limit loads of a plate with an elliptical hole under uniaxial loading and with a circular hole under biaxial loading in plane stress condition are calculated. The analytical results are then compared with Finite element calculations and the correlation between them is discussed. V.N. Skopinsky (2006) presented work on structural modeling and stress analysis of nozzle connections in ellipsoidal heads subjected to external loadings. Timoshenko shell theory and the finite element method are used. Results showed that it is necessary to pay more attention to the effective stresses in the shells in these loading cases. The effect of the stress concentration is more significant than in the case of internal pressure loading, i.e., there is an appreciable increase of the maximum stresses for shells in the intersection region even at the small level of nominal stresses. Non-radial and offset connections have significantly non-uniform distribution of the effective stresses on the intersection curve between the nozzle and the head. Amran Ayob (2006) presented work on stress field at the intersection of a radial nozzle attached to a torispherical crown of a cylindrical vessel. The finite element method was used to determine the stress field at the intersection. It is concluded that for all load categories, high stresses occurred at the vessel nozzle junction where there is a severe geometric discontinuity. Nozzle thrust load gives the highest stress while torsional moment gives the lowest stress. When torque is one of the combining loads, a circular interaction is proposed. For other load combinations a linear relation is proposed. | ||||||
| A.B. Smetankin (2006) worked on the structural modeling and stress analysis of nozzle connections in ellipsoidal heads subjected to external loadings. Timoshenko shell theory and the finite element method are used. The features of the structural modeling of ellipsoid-cylinder shell intersections, numerical procedure and SAIS special-purpose computer program are discussed. A parametric study of the effects of geometric parameters on the maximum effective stresses in the ellipsoid-cylinder intersections under loading was performed. The results of the stress analysis and parametric study of the nozzle connections are presented. Results show that it is necessary to pay more attention to the effective stresses in the shells in these loading cases. Although the stresses due to the external loadings are secondary stresses with respect to primary stresses from the internal pressure, these stresses should be taken into consideration in a complete stress analysis for nozzle connections of a pressure vessel. E. Oterkus (2007) presented work on semianalytical solution method for determining the stress and deformation fields in a thin, laminated-composite cylindrical shell with an elliptical cutout. The analysis includes the effects of cutout size, shape, and orientation; non-uniform wall thickness; oval cross-sectional eccentricity; and loading conditions. The loading conditions include uniform tension, uniform torsion, and pure bending. The analysis approach is based on the principle of stationary potential energy and uses Lagrange multipliers to relax the kinematic admissibility requirements on the displacement representations through the use of idealized elastic edge restraints. G. H. Rahimi (2007) presented work on limit analysis of cylindrical shell with a circular opening under the action of combined axial force and bending moment at the ends by using finite element methods and the approximate analytical lower-bound limit load of the weakest section of the cylinder. It is presented that the lower bound limit load using the weakest cross-section method is independent of shape, length and axial location of opening and is only a function of weakest cross-section of cylinder. R. A. Alashti (2007, 2008) presented work on the plastic limit load of cylindrical shells with openings subject to combined bending moment and axial force is found through finite element method. The effects of various parameters such as the geometrical ratios of shell and the shape, size, axial and angular position of the opening on the limit load of cylindrical shells under various loading conditions are studied with circular and elliptical opening. J. Fang and Q.H. Tang (2008) presented the work on a comparative study of strength behavior for cylindrical shell intersections with and without pad reinforcement under out-of-plane moment loading on nozzle. Three pairs of full-scale test vessels with different d/D ratios were designed and fabricated for testing and analysis. The results indicated that the effect of pad reinforcement on decreasing maximum elastic stress and increasing plastic limit load is obviously effective. B. Suresh (2008) presented analysis of stresses around an elliptical cutout/inclusion in a filament wound fiber reinforced plastic pressure vessel for different axis ratio (k) using ANSYS. In conclusion they found that in case of laminated composite cylindrical shell with cutout, the maximum stress occurs on the bottom surface of layer 1 at η = 90° for all the values of axis ratio selected. In case of laminated composite cylindrical shell with inclusion, as the axis ratio (k) increases the maximum stress near the cutout region decreases. The effect of axis ratio is quite significant in case of laminated composite cylindrical shell with cutout and the maximum stress increases with increase in axis ratio (k). The effect of axis ratio for the case of laminated composite cylindrical shell with inclusion is not as significant as that of cutout and the maximum stress decrease with increase in axis ratio. M. Javed Hyder (2008) presented optimize the location and size of opening (hole) in a pressure vessel cylinder using ANSYS. Analysis is performed for three thick-walled cylinders with internal diameters 20, 25 and 30 cm having 30 cm height and wall thickness of 20 mm. It is observed that as the internal diameter of cylinder increases the Von Misses stress increases. Optimization of hole size is carried out by making holes having diameter of 4, 8, 10, 12, 14, 16 and 20 mm located at center in each of the three cylinders, and it is observed that initially Von Misses stress decreases and then become constant with hole size. | ||||||
| Lei Zu (2009) presented work on filament-wound isotensoids are mostly based on geodesic winding. However, the geometry of geodesics is certainly limiting the available design space. A typical restriction is the inability to create isotensoids with unequal openings at both ends. A simplified method for designing isotensoid pressure vessels with unequal polar opening is outlined, using non-geodesic trajectories. Firstly we present the non-geodesic equations on general shells of revolution. Next, a direct relation among the shell curvatures, roving force, internal pressure and slippage coefficient, as a basis for determining non-geodesics-based isotensoid shapes, is provided. The governing equations for specifying meridian profiles are derived in terms of the slippage coefficient. The meridian profiles of nongeodesics- based isotensoid corresponding to various opening radii and slippage coefficients are determined, and theperformance factors of the obtaining domes are calculated to demonstrate the effect the application of non-geodesics has on the structural efficiency. A stable and easily accessible solution procedure is proposed to determine the slippage coefficients fulfilling the winding requirements. Results show that the present method is suitable for the design of isotensoid structures with unequal polar openings. Results also indicate that the non-geodesics-based isotensoid domes show better performance than the geodesic–isotensoid. Duncan Camilleri (2009) presented work on the shakedown behavior of a thin cylinder subject to constant pressure and cyclic thermal loading is described by the well known Bree diagram. The shakedown and ratcheting behavior of a thin cylinder, a thick cylinder and a thick cylinder with a radial crosshole is investigated by inelastic finite element analysis. The DBA of this component can therefore be achieved by reference to standard thick cylinder theory solutions for the global failure mechanisms (limit load and elastic shakedown load), augmented by Stress Concentration Factor data for peak stress and hence fatigue evaluation. S. Laczek (2010) presented the work on elastic-plastic analysis of the stress-strain state in the vicinity of a hole in a thickwalled cylindrical pressure vessel. Using finite element calculations, different failure criteria are proposed to aid design and control of high-pressure vessels with piping attachments. It is reported that the local stress distribution near the hole results in a specific failure of the vessel, the vessel unloading can cause local reverse plasticity, which leads to plastic shakedown in the small zone and then to progressive ductile fracture in this zone. The summary of the literature is presented in Table I | ||||||
| B. Literature review of flat plat with opening: | ||||||
| In above literature it is seen that most of the author used critical methods to study the effect of the stress distribution around the openings on cylinder and spherical shell. To avoid the complications in study of stress distribution around opening some researchers used the flat plate with uniaxial and biaxial loading. Following literature review describes the study done by researchers in on flat plate with openings. | ||||||
| A. Tafreshi (1995) presented work on stress analysis of a series of thick, wide, flat plates with oblique holes subjected to uniaxial tension and out-of-plane bending has been carried out using the finite element method (FEM), and in some cases the boundary element method (BEM). Different plate thickness-hole diameter ratios, angles of hole obliquity and orientation have been considered to provide stress concentration factors at such holes. The work covers plate thickness-hole diameter ratios from 1.3 to 3.0, hole obliquity angles from 0 to 60º and orientation of the major axis of the surface ellipse relative to the applied load direction of 0 to 90º. The results for uniaxial tension have been compared with those determined using the photoelastic frozen-stress technique in order to verify the finite element models before proceeding to the bending cases, which provide new data. They concluded that FE results and experimental results were within 8.6 per cent, which showed good agreement. For α = 60°, for example, the accuracy of FE results was very poor and the differences between the FE results and experimental results were about 20-30 per cent. E.S. Folias (1999) presented work on the theory of thin shell structures, a failure criterion is presented which one may use to predict, analytically, catastrophic failures, or unzipping, in cylindrical pressurized vessels, based on the fracture toughness profile K obtained from tests carried out on flat plates of the same material and thickness. These test results are plotted as a function of the characteristic ratio (h/c), where h represents the specimen thickness and c one-half of the crack length. Comparison with carefully controlled experimental data substantiates its validity and its potential use. The advantage of such an approach is that considerable amount of time and money can be saved. Dwight Snowberger (2008) study the effect an elliptical hole has on the stress distribution of a flat plate as the sharpness of the ellipse increases from a circle to a narrow crack for the specific geometry of a flat plate that is 10x20 inches and a 2-inch long diameter elliptical hole. Two element types will be compared, which are the 4-noded quad (ANSYS Plane42) and the 8-noded quad (ANSYS Plane82). Finally, the equations for a flat plate with a circular hole and an elliptical hole will be compared for the case when the elliptical hole is a circle in order to show that the results are similar enough that the elliptical equations can be used for the case when the ellipse becomes a circle. | ||||||
| The summary of the literature is presented in Table II | ||||||
| C. Literature review of Stress Concentration Factor around openings: | ||||||
| The maximum stress will be much larger if there is opening in the shell than in the case where there is no penetration. This causes the rise in the stress distribution around the opening to calculate this stress rising around hole the stress concentration factor where mostly used in practice. This section describes the work done by researchers on stress concentration factor at openings in pressure vessels. | ||||||
| J.M. Kihiul (1995) presented work on the hoop stress and stress concentration factor distributions in a closed ended thick-walled cylinder with a chamfered cross bore under internal pressure. The finite element method of stress analysis is used for this study. The effect of changing chamfer angle, for a fixed chamfer size, on the hoop stress and stress concentration factor distributions was investigated. The optimum chamfer angle and chamfer length for any cylinder configuration was established. The study revealed that adding chamfers to cross bores causes a, redistribution and reduction of the stresses attained in internally pressurized thick-walled cylinders. For thick cylinder of a given thickness ratio and cross bore diameter, it was observed that the stresses were a minimum at a specific chamfer angle and size. K. B. Mulchandani (1995) presented work on the problem of determining the opening mode stress intensity factor (SIF) from the photoelastic isochromatic fringe pattern associated with a surface crack located in the ligament region between two radial nozzle-cylinder junctions of pressure vessel. The objective is to determine the influence of geometry, size and location of the surface flaw with respect to the radial nozzles. A new method suited to the analysis of photoelastic data obtained from a single isochromatic fringe loop to extract the SIF. Further, the extent of reduction in SIF values was found to be dependent upon the geometry, size and relative location distance of surface flaw with respect to the nozzle centerline. K. Magnucki (2002) presented work on the problem of stress concentration in a cylindrical pressure vessel with ellipsoidal heads subject to internal pressure. At the line, where the ellipsoidal head is adjacent to the circular cylindrical shell, a shear force and bending moment occur, disturbing the membrane stress state in the vessel. It is reported that the degree of stress concentration depends on the ratio of thicknesses of both the adjacent parts of the shells and on the relative convexity of the ellipsoidal head, with the range for radius-to-thickness ratio between 75 and 125. The stress concentration was analytically described and, afterwards, the effect of these values on the stress concentration ratio was numerically examined. S. Schindler (2003) worked on the widely used stress concentration factors for the radial nozzle to spherical shell connection given in the British Standard Specification for Unfired fusion welded pressure vessels [British Standard Specification for Unfired fusion welded pressure vessels, 2000; Welding Research Bulletin, 1963] are examined and contrasted with recent Finite Element Method simulations. Deviations are noted and, as far as possible, explained. Example graphs showing the possible improvement, with regard to accuracy, and also with regard to other improvements related to cyclic fatigue calculations. | ||||||
| P Makulsawatudom (2004) presented work on elastic stress concentration factors (SCFs) for internally pressurized thick cylindrical vessels with radial and offset circular and elliptical crossholes. Three forms of intersection between the crosshole and main bore are considered: plain chamfered and blend radius. The minimum SCF was found to occur for the plain intersection configuration, with the peak stress at the crotch corner between the main bore and crosshole on the longitudinal plane of symmetry. Incorporating a chamfer or blend radius at the intersection reduces the stress concentration at the main bore but introduces higher peak stress elsewhere in the chamfer or blend region. The finite element analysis parametric investigation confirmed the well-known result that the SCF is reduced when the intersection between the crosshole and main bore surface has an elliptical profile. The radial elliptical crosshole reduces the SCFs significantly but in general leads to greater manufacturing cost. The offset circular crosshole, which is cheaper and easier to construct, also reduces the stress concentration effect although the reduction is less than that of an elliptical crosshole. The investigation considered two relatively small openings, typical of instrumentation tapping, bursting caps or fluid entry/exit ports in thick high-pressure vessels. Overall, the stress concentration effect was greater for the smaller hole, although the difference was only about 5%. When the effect of crosshole end-cap thrust was considered, the SCF did not change significantly but was slightly alleviated. | ||||||
| You-Hong Liu (2004) presented work on Limit pressures and corresponding maximum local membrane Stress Concentration Factors (SCF) for two orthogonally intersecting thin-walled cylindrical shells subjected to internal pressure. The local membrane SCF at the intersections of two cylindrical shells subjected to the limit pressure load is calculated by elastic thin shell theoretical solutions. 3D finite element method (using ANSYS) in which the material is elastic-perfectly plastic is used. From result they found that the local membrane SCF decreases significantly as t/T increases, and decreases little as d/ √DT decreases when D/T is fixed. The local membrane SCF increases significantly as D/T increases, and varies little as d/ √DT increases when t/T is constant. | ||||||
| E.A. de Carvalho (2005) presented work on stress concentration factors for an internally pressurized cylinder containing a radial U-notch along its length. This work studies the cases where the external to internal radius ratio (ψ) is equal to 1.26, 1.52, 2.00, and 3.00 and the notch radius to internal radius ratio (?) is fixed and equal to 0.026. The Unotch depth varies from 0.1 to 0.6 of the wall thickness. Results are also presented for a fixed size semi-circular notch. Hoop stresses at the external wall are presented, showing regions where the stress matches the nominal one and the favorable places to install strain sensors. The finite element method is used to determine the stress concentration factors (Kt) for the above described situations and for a special case where a varying semi-circular notch is present with ψ = 3.00. This notch depth varies from 0.013 to 0.3 of the wall thickness. It is pointed out that even relatively small notches introduce large stress concentrations and disrupt the hoop stress distribution all over the cross section. Results are also compared to an example found in the literature for semi-circular notches and Kt curves for both cases present the same shape. | ||||||
| J.M. Kihiu (2006) developed a three-dimensional finite element computer program to establish the stress distributions and stress concentration factors (SCFs) in chamfered cross-bored cylinders under internal pressure. In optimal chamfered cylinders with thickness ratio between 2.25 and 3, the SCF was found to increase with decrease of thickness ratio. Thick cylinders were found to be more suited to chamfering than thin cylinders. The resulting data in this work provides a useful and quick design tool. Kh. Fuad (2007) analyzed the stress concentration factor (SCF) of adjacent holes in a spherical pressure vessel by considering a thin plate undergoing hydrostatic stresses. He investigated the SCF of various adjacent holes configurations in a spherical pressure vessel using finite element analysis based on the VonMises stresses. Various arrangements of adjacent holes are investigated. The results showed that the decreasing of L/d will affect the increasing of SCF, while for the case of five adjacent holes configuration, the increasing of d/t doesn?t make any significant effect to the increasing of SCF. Dwight Snowberger (2008) presented work on the effect of an elliptical hole on the stress distribution of a flat plate. The analysis is carried out using ANSYS for a flat plate with a circular hole and an elliptical hole and concluded that the elliptical equations can be used for the case when the ellipse becomes a circle. M. Qadir (2009) presented work on SCF analysis of a pressurized vessel–nozzle intersection with wall thinning damage. Among the significant observations was the systematic rise in the SCF value with an increase in the diameter ratio d/D, for a specified vessel diameter-thickness ratio D/T. It was also observed that for a specified d/D ratio, the SCF value increases as the D/T ratio is increased. The summary of the literature is presented in Table III | ||||||
IV. SUMMARY OF THE LITERATURE REVIEW |
||||||
| Form the literature review it is seen that ASME and other codes are providing solutions for more general cases and requires higher factor of safety. Also limit load and stress concentration formulae are not available for non standard shapes and intersections and geometrical discontinuity. The code does not consider for openings in thin pressure vessels but some researchers have shown that openings in thin pressure vessel are changing stress concentration value by considerable amount. | ||||||
| Most of the researchers have worked on thick pressure vessels, few researchers have worked in thin-pressure vessels and there is scope in studying the openings in thin pressure vessels. Some researchers studied the effect of discontinuity in flat plate with the hole/opening in uniaxial and biaxial stresses which gives the basic idea about the stress and loading effect at point of discontinuity. Most of them have used parametric method to study the effect of the different design parameter like thickness, diameter of the cylinder/shell, opening size. The analytical methods used by some researchers basically used the flat plate theory and stress concentration factor or elastic-plastic limit load study. Most of the researchers have used experimental method in which the results are obtained directly. | ||||||
| From above discussion it is cleared that study of the effect of change in size, position, location of the openings in pressure vessel to study the stress concentration is essential. The position and location of the opening on cylinder is not studied in past by researchers and there is no code provision for such design. For such problems codes are suggesting use of DBA (Design by analysis) that includes non linearity. Majority of research have preferred design by analysis than design by code. This approach is helpful in simulating the exact mode of failure in pressure vessel. From the above literature it is also seen that the finite element method was used by most of the researchers to compare the analytical and experimental results. So it is clear that finite element method is the efficient method to use for simulating the effect. | ||||||
V. CONCLUSION |
||||||
| Stress Concentration is one of the important factors to be studied in the pressure vessels openings. A review of the literature related to the stress concentration at openings in pressure vessels is presented. Majority of the researchers have worked on thick cylinders and there is a scope in working for thin cylinders. Also the effect of end covers on the position and size of the openings needs to be studied. | ||||||
Tables at a glance |
||||||
|
||||||
References |
||||||
|