International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Menu

ISSN ONLINE(2319-8753)PRINT(2347-6710)

All submissions of the EM system will be redirected to Online Manuscript Submission System. Authors are requested to submit articles directly to Online Manuscript Submission System of respective journal.

Theory of Fuzzy Soft Matrix and its Multi Criteria in Decision Making Based on Three Basic t-Norm Operators

Md. Jalilul Islam Mondal1 , Dr. Tapan Kumar Roy2
  1. Research Scholar, Dept. of Mathematics, BESUS, Howrah-711103 , W.B., India
  2. Professor, Dept. of Mathematics, BESUS, Howrah-711103 , W.B. India
Related article at Pubmed, Scholar Google

Visit for more related articles at International Journal of Innovative Research in Science, Engineering and Technology

International Journal of Innovative Research in Science, Engineering and Technology

Abstract

The purpose of this paper is to put forward the notion of fuzzy soft matrix theory and some basic results. In this paper, we define fuzzy soft matrices and some new definitions based on t-norms with appropriate examples .Lastly we have given an application in decision making based on different operators of t-norms.

Keywords
Soft sets, fuzzy soft matrices, operators of t-norms.

INTRODUCTION
Most of our traditional tools for formal modeling, reasoning, and computing are crisp, deterministic, and precise in character. However, in real life, there are many complicated problems in engineering, economics, environment, social sciences medical sciences etc. that involve data which are not all always crisp, precise and deterministic in character because of various uncertainties typical problems. Such uncertainties are being dealing with the help of the theories, like theory of probability, theory of fuzzy sets, theory of intuitionistic fuzzy sets, theory of interval mathematics and theory of rough sets etc. Molodtsov [1] also described the concept of “Soft Set Theory” having parameterization tools for dealing with uncertainties. Researchers on soft set theory have received much attention in recent years. Maji and Roy [3,4] first introduced soft set into decision making problems. Maji et al.[2] introduced the concept of fuzzy soft sets by combining soft sets and fuzzy sets. Cagman and Enginoglu [5] defined soft matrices which were a matrix representation of the soft sets and constructed a soft max-min decision making method. Cagman and Enginoglu [6] defined fuzzy soft matrices and constructed a decision making problem. Borah et al.[7] extended fuzzy soft matrix theory and its application. Maji and Roy [8] presented a novel method of object from an imprecise multi-observer data to deal with decision making based on fuzzy soft sets. Majumdar and Samanta[9] generalized the concept of fuzzy soft sets. In this paper, we have introduced some operators of fuzzy soft matrix on the basis of t-norms . We have also discussed their properties . Finally we have given an application in decision making problem on the basis of t-norms operators .

DEFINITION AND PRELIMINARIES
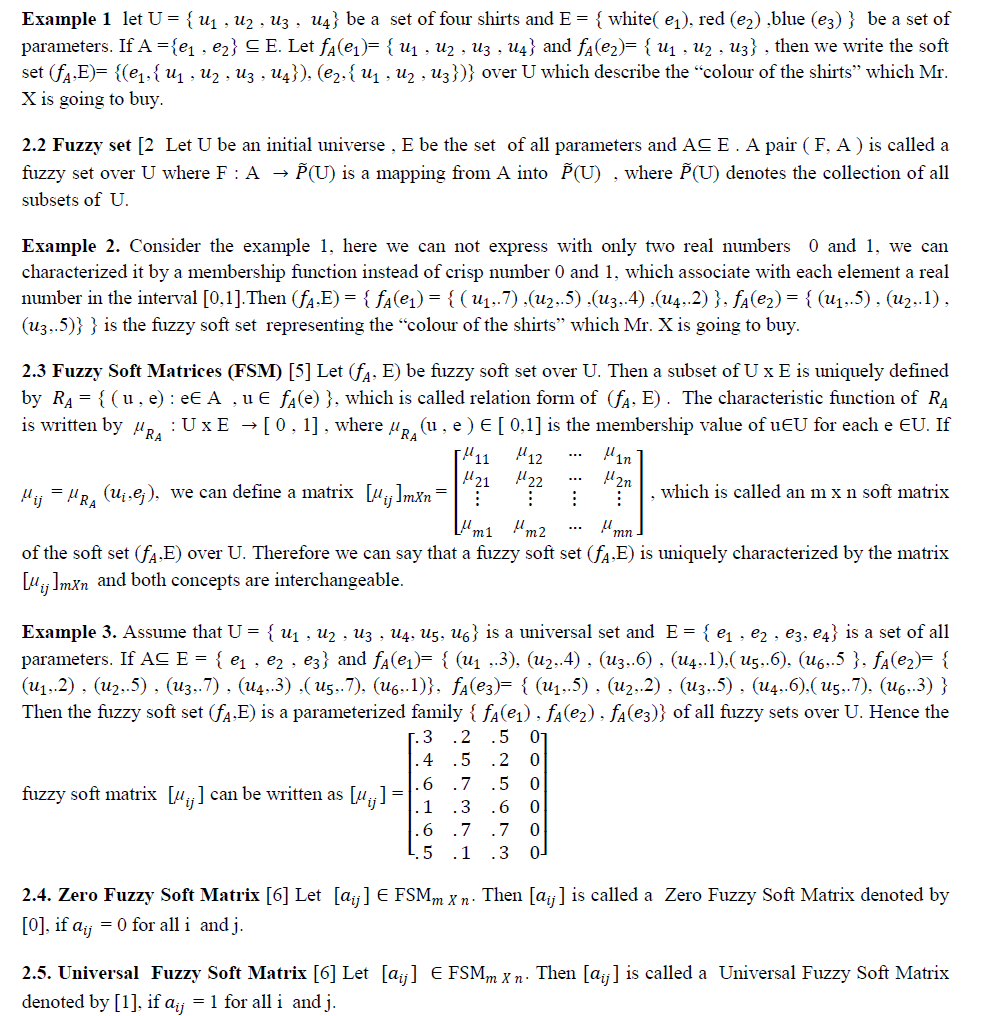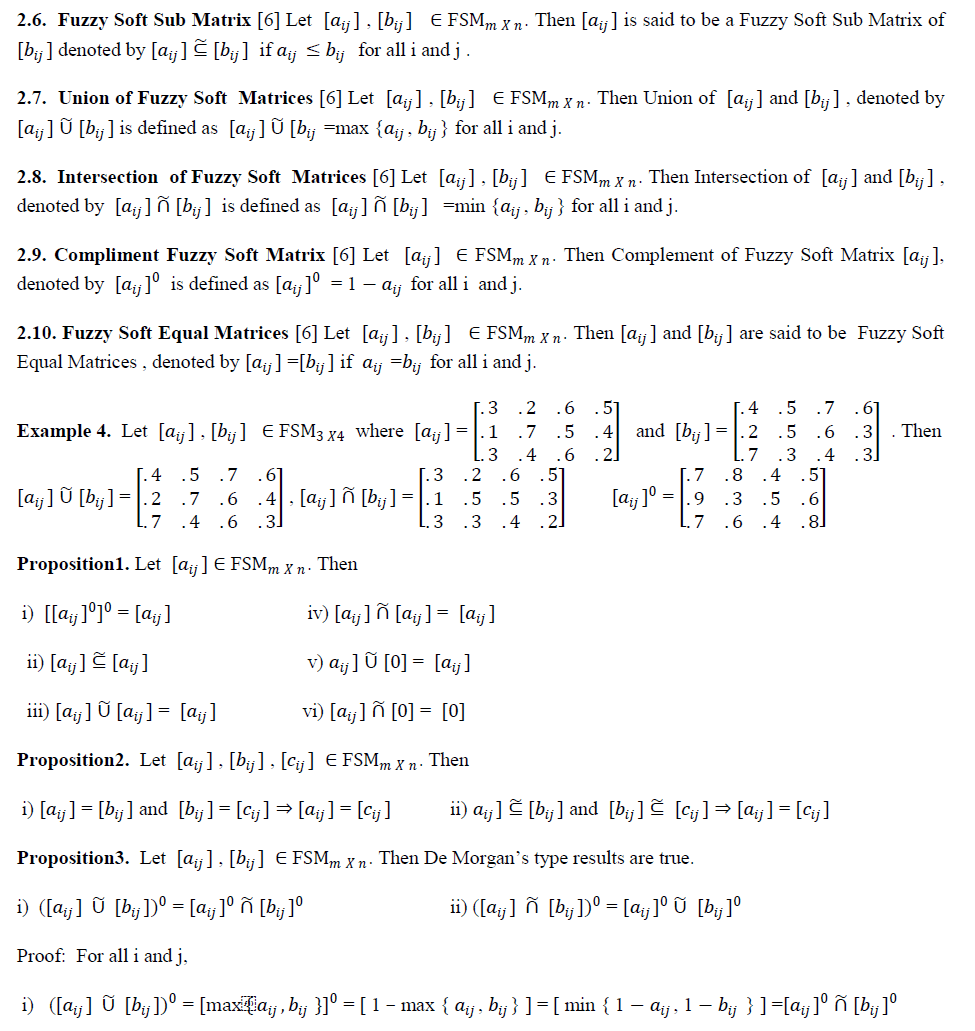
image
image
image
image
image
image
image
image

CONCLUSION
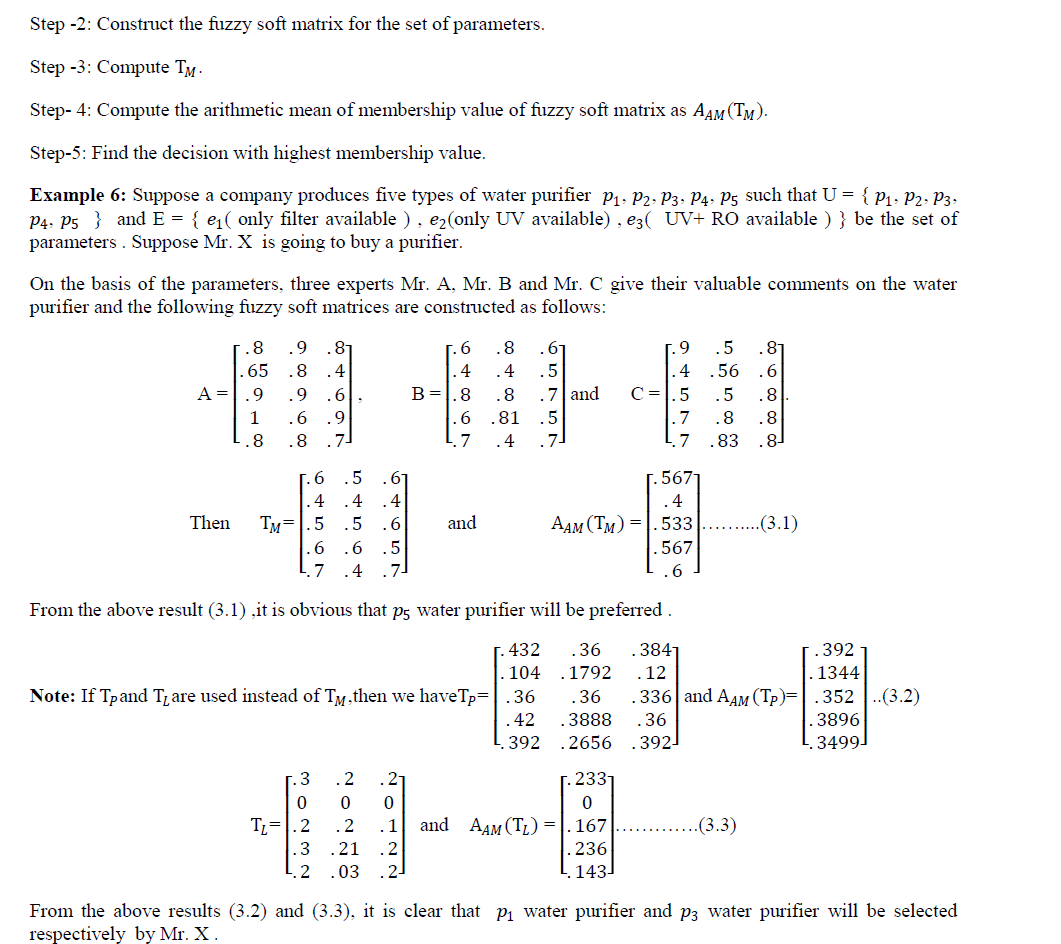
In this paper, we proposed fuzzy soft matrices and defined different types of fuzzy soft matrices. We have also given some definitions based on t-norm with examples and some properties with proof . Some operators on t-norm are also given. Finally, we extend our approach on t-norms in application of decision making problems. We have shown that decisions are different for different methods on same application. Our future work in this regard is to find other methods whether the notions in this paper yield fruitful result.

References
  1. D. Molodtsov, “Soft set theory – first result”, Computers and Mathematics with Applications 37(1999), pp. 19-31 .
  2. P. K. Maji, R. Biswas and A. R. Roy, “Fuzzy Soft Sets”, Journal of Fuzzy Mathematics , 9(3), ( 2001), pp.589 – 602.
  3. P.K.Maji , R. Biswas and A.R.Roy, “An application of soft sets in a decision making problems”, Computer and Mathematics with Applications, 44(2002), pp. 1077-1083.
  4. P.K.Maji , R. Biswas and A.R.Roy, “Soft Set Theory”, Computer and Mathematics with Applications, 45(2003), pp. 555-562 .
  5. Naim Cagman, Serdar Enginoglu, “Soft matrix theory and its decision making”, Computers and Mathematics with Applications 59(2010), pp. 3308- 3314.
  6. N. Cagman and S. Enginoglu, “Fuzzy soft matrix theory and its application in decision making”, Iranian Journal of Fuzzy Systems, 9(1), (2012), pp. 109-119.
  7.   Manas Jyoti Borah, Tridiv Jyoti Neog, Dusmanta Kumar Sut, “Fuzzy soft matrix theory and its decision making” , IJMER, 2(2) (2012), pp.121-127.
  8.   P. K.Maji , A. R. Roy, “A fuzzy soft set theoretic approach to decision making problems”, Journal of Computational and Applied Mathematics, 203(2007), pp. 412 – 418.
  9.   P. Majumdar , S.K.Samanta, “Generalized fuzzy soft sets”, Computers and Mathematics with Applications, 59(2010), pp. 1425-1432.
  10. James J. Buckley, Esfandiar Eslami, “An Introduction to Fuzzy Logic and Fuzzy Sets”, Physica-Verlag, Heidelberg ,New York(2002).