ISSN: 2320-2459
ISSN: 2320-2459
Govt Arts College for Women (Autonomous), Pudukkottai, Tamil Nadu-622 001, India.
Received Date: 25/09/2015; Accepted Date: 25/04/2016; Published Date: 28/04/2016
Visit for more related articles at Research & Reviews: Journal of Pure and Applied Physics
Detergent is an aggregation of surfactants, builders, fillers by enabling the solution to wet a surface quickly and effectively. It also emulsifies oily soils and keep them, bleaches, dyes, enzymes and several other ingredients. Surfactants cleanses suspended and dispersed so they do not settle back on the surface. To achieve superior cleaning performance, other compounds like builders and fillers are added to surfactants. Sodium dodecyl sulphate is an anionic surfactant, primary ingredient added to detergents and cleaning products. In the present investigation, the molecular interactions of SDS are studied through the addition of fillers sodium chloride and sodium sulphate. The important usage of NaCl and Na2SO4 are in the manufacture of detergents. Sodium sulphate is a very cheap material, consuming approximately about 50% of world production. It helps in “leveling”, reducing negative charges on fibers so that dyes can penetrate evenly on fabrics. Similarly sodium chloride also serves as effective filler when added to SDS. The efficiency of these SDS and fillers in detergent action can be analyzed by the thermo dynamical study using ultrasonic method with the measurement of ultrasonic velocity, viscosity and density. Using the measured values, thermo dynamical parameters like internal pressure, free volume, osmotic pressure, Δπi, Gibb’s free energy, molar cohesive energy were evaluated for aqueous SDS with fillers at different temperatures. Ultrasonic study of the aqueous solutions reveals some information regarding internal pressure which is a single factor appears to vary due to the internal cohesive forces resultant from attractive and repulsive forces between the molecules. It measures the molecular cohesion and instantaneous volume derivative of cohesive energy associated with an isothermal expansion of solutions. The internal pressure of hydrogen bonded liquids (water) is large as compared to nonhydrogen bonded liquids. Hence it can be used for studying molecular association of hydrogen bonding. Similarly free volume is one of significant factor in explaining the free space and its dependent properties have close connection with molecular structure and it may show features about various interactions. It seems to be conditioned by repulsive forces whereas internal pressure is sensitive to attractive forces. Gibb’s free energy is the energy associated with a chemical reaction that can be used to do work. Molar cohesive energy is arising due to the mutual attractiveness of molecules. Osmotic pressure is the minimum pressure which needs to be applied to a solution to prevent the inward flow of solvent molecules across a semipermeable membrane. Various interactions of SDS with fillers are explained in terms of above parameters and also the relationships πi=π0+Am2+Bm for internal pressure and Vf=Vf0+Cm2+Dm for free volume were also verified. Thecoefficients A, B and C, D for the above equation have been calculated at different temperatures. Δπi gives an idea about the effect of cohesive forces in ion-solvent interaction. It is understood that sensitive information regarding the cohesive forces is well obtained by the data Δπi instead of the coefficients of the above equation.
SDS, Sodium chloride, Sodium sulphate, Molar cohesive energy, Internal pressure, Free volume, Gibb’s free energy.
A laundry detergent composition generally comprises six groups of substances: surfactants, builders, enzymes, bleaching agents, fillers and other minor additives such as dispersing agents, fabric softening clay and optical brighteners [1]. Detergents and household, personal products account for over half the use of surfactant [2]. Hence knowledge about the surfactant nature with other ingredients is the driving force for the detergent usage and their related industrial applications. In this article, the studies of molecular interactions present in the surfactant solutions combined with the fillers were done by calculating the Thermodynamical parameters and their results were discussed. The anionic surfactant sodium dodecyl sulphate taken under study is used in greater volume than any other groups due to their ease and low cost of manufacture. Detergent fillers are additives that are added to detergents to improve the cleansing performance. The objective of adding fillers to detergents is to make detergents fluid or to turn the fluidized detergents in powder form. The parameters such as ultrasonic velocity, density, viscosity and other related Thermodynamical parameters provide better insight into intermolecular interactions. The investigation is carried out to calculate the Thermodynamical parameters of sodium dodecyl sulphate with the addition of fillers and to interpret the results of them (Table 1).
| Temperature | Molality(mm) | Internal Pressure(*108 N/m2) | Free Volume(*10-8 m3) | Osmotic pressure | |||
|---|---|---|---|---|---|---|---|
| 298 K | NaCl | Na2SO4 | NaCl | Na2SO4 | NaCl | Na2SO4 | |
| 0 | 27.8756 | 27.8756 | 1.7193 | 1.7193 | 0.2231 | 0.2231 | |
| 2 | 27.5480 | 27.5539 | 1.7775 | 1.7763 | 0.0892 | 0.0889 | |
| 4 | 27.6697 | 27.5847 | 1.7540 | 1.7702 | 0.1783 | 0.1334 | |
| 6 | 27.6966 | 27.7351 | 1.7499 | 1.7404 | 0.2674 | 0.2666 | |
| 8 | 27.7230 | 27.7292 | 1.7455 | 1.7414 | 0.356 | 0.3554 | |
| 10 | 27.7128 | 27.7102 | 1.7480 | 1.7450 | 0.444 | 0.4436 | |
| 12 | 27.8241 | 27.8254 | 1.7301 | 1.7240 | 0.5325 | 0.5305 | |
| 14 | 27.9212 | 27.8997 | 1.7147 | 1.7135 | 0.6211 | 0.6175 | |
| 308 K | 0 | 25.4539 | 25.4539 | 2.4601 | 2.4601 | 0.2306 | 0.2306 |
| 2 | 25.2589 | 25.2763 | 2.5267 | 2.3348 | 0.0922 | 0.0919 | |
| 4 | 25.1526 | 25.2292 | 2.5595 | 2.4028 | 0.1843 | 0.1378 | |
| 6 | 25.3200 | 25.3334 | 2.5116 | 2.4445 | 0.2764 | 0.2756 | |
| 8 | 25.4856 | 25.4866 | 2.4671 | 2.5205 | 0.3679 | 0.3673 | |
| 10 | 25.4911 | 25.5298 | 2.4654 | 2.5829 | 0.4589 | 0.4585 | |
| 12 | 25.5434 | 25.6333 | 2.4520 | 2.6682 | 0.5504 | 0.5483 | |
| 14 | 25.5734 | 25.7396 | 2.4482 | 2.3926 | 0.642 | 0.6382 | |
| 318 K | 0 | 22.4784 | 22.4784 | 3.9129 | 3.9129 | 0.2381 | 0.2381 |
| 2 | 23.6586 | 23.6792 | 3.3550 | 3.3453 | 0.0952 | 0.0949 | |
| 4 | 23.6075 | 23.6637 | 3.3784 | 3.3516 | 0.1903 | 0.1423 | |
| 6 | 23.5976 | 23.6474 | 3.3824 | 3.3566 | 0.2854 | 0.2845 | |
| 8 | 23.7156 | 23.6963 | 3.3358 | 3.3358 | 0.3799 | 0.3792 | |
| 10 | 23.8258 | 23.7348 | 3.2929 | 3.3217 | 0.4738 | 0.4734 | |
| 12 | 23.8221 | 23.8100 | 3.2950 | 3.2894 | 0.5683 | 0.5661 | |
| 14 | 23.8643 | 23.9441 | 3.2784 | 3.2329 | 0.6628 | 0.659 | |
| 328 K | 0 | 21.5276 | 21.5276 | 4.8451 | 4.8451 | 0.2456 | 0.2456 |
| 2 | 21.8923 | 21.9034 | 4.6040 | 4.5946 | 0.0982 | 0.0979 | |
| 4 | 21.8826 | 22.0608 | 4.6139 | 4.4695 | 0.1963 | 0.1468 | |
| 6 | 21.9542 | 22.1747 | 4.5697 | 4.4010 | 0.2944 | 0.2935 | |
| 8 | 21.8970 | 22.2975 | 4.6043 | 4.3200 | 0.3918 | 0.3912 | |
| 10 | 21.9099 | 22.4040 | 4.6011 | 4.2744 | 0.4887 | 0.4883 | |
| 12 | 21.9775 | 22.5524 | 4.5648 | 4.1762 | 0.5861 | 0.5839 | |
| 14 | 21.9994 | 22.5567 | 4.5526 | 4.1655 | 0.6837 | 0.6797 | |
Table 1: Values of internal pressure, free volume and osmotic pressure of aqueous sodium dodecyl sulphate combined with sodium chloride and sodium sulphate at different temperatures.
AnalaR grade samples of Sodium dodeyl sulphate, sodium chloride and sodium sulphate were used for the present investigation. Solutions of sodium dodecyl sulphate (10 mm) added with fillers sodium chloride and sodium sulphate at different concentrations (2 mm to 14 mm) was prepared. Ultrasonic velocity is measured using an ultrasonic interferometer (Mittal F-81D) with fixed frequency 2 MHz. Density is measured using specific gravity bottles at various temperatures with constant temperature bath. Viscosity is measured using Ostwald viscometer.
Theoretical formulations
The following Thermodynamical parameters were calculated from following relations using the velocity, density and viscosity values (Table 2).
| Temperature | Molality(mm) | Molar Cohesive energy(*104 KJ mol-1) | Gibb’s Free energy(*10-21 KJ mol-1 ) | Δπi | |||
|---|---|---|---|---|---|---|---|
| 298 K | NaCl | Na2SO4 | NaCl | Na2SO4 | NaCl | Na2SO4 | |
| 0 | 5.0384 | 5.0384 | 5.6148 | 5.6148 | 0.7435 | 0.7435 | |
| 2 | 4.9847 | 4.9857 | 5.3996 | 5.5773 | -0.3276 | -0.3216 | |
| 4 | 5.0068 | 4.9915 | 5.4231 | 5.4697 | -0.2059 | -0.2908 | |
| 6 | 5.0102 | 5.0204 | 5.3973 | 5.4047 | -0.1790 | -0.0140 | |
| 8 | 5.0142 | 5.0195 | 5.3702 | 5.3518 | -0.1525 | -0.1463 | |
| 10 | 5.0115 | 5.016 | 5.3374 | 5.2148 | -0.1628 | -0.1653 | |
| 12 | 5.0273 | 5.0361 | 5.34365 | 5.1452 | -0.0515 | -0.0501 | |
| 14 | 5.0410 | 5.0447 | 5.34360 | 5.3381 | 0.0455 | 0.0241 | |
| 308 K | 0 | 4.6316 | 4.6316 | 4.5665 | 4.5665 | 0.4987 | 0.4987 |
| 2 | 4.5887 | 4.5905 | 4.3507 | 4.5573 | -0.1950 | -0.1775 | |
| 4 | 4.5678 | 4.5817 | 4.2633 | 4.4368 | -0.3013 | -0.2246 | |
| 6 | 4.5960 | 4.6016 | 4.3093 | 4.3779 | -0.1338 | -0.0120 | |
| 8 | 4.6221 | 4.6281 | 4.3343 | 4.2732 | 0.0317 | 0.0327 | |
| 10 | 4.6232 | 4.6371 | 4.3253 | 4.1886 | 0.0371 | 0.0759 | |
| 12 | 4.6311 | 4.6553 | 4.3338 | 4.0904 | 0.0894 | 0.1794 | |
| 14 | 4.6319 | 4.6702 | 4.3284 | 4.3791 | 0.1195 | 0.2857 | |
| 318 K | 0 | 4.0998 | 4.0998 | 3.3185 | 3.3185 | -0.7750 | -0.7750 |
| 2 | 4.3157 | 4.3200 | 3.7752 | 3.7727 | 1.1802 | 1.2252 | |
| 4 | 4.3054 | 4.3174 | 3.7281 | 3.7446 | 1.1291 | 1.2097 | |
| 6 | 4.3038 | 4.3157 | 3.7163 | 3.7188 | 1.1192 | 1.1934 | |
| 8 | 4.3230 | 4.3247 | 3.7206 | 3.7145 | 1.2372 | 1.2423 | |
| 10 | 4.3409 | 4.3303 | 3.7449 | 3.7031 | 1.3473 | 1.2808 | |
| 12 | 4.3399 | 4.3447 | 3.7314 | 3.7156 | 1.3437 | 1.3560 | |
| 14 | 4.3470 | 4.3702 | 3.7233 | 3.7617 | 1.3859 | 1.4901 | |
| 328 K | 0 | 3.9438 | 3.9438 | 2.9056 | 2.9056 | -0.4114 | -0.4114 |
| 2 | 4.0119 | 4.0150 | 3.07593 | 3.0597 | 0.3646 | 0.3758 | |
| 4 | 4.0085 | 4.0445 | 3.04418 | 3.1088 | 0.3549 | 0.5332 | |
| 6 | 4.0213 | 4.0654 | 3.05823 | 3.138 | 0.4266 | 0.6471 | |
| 8 | 4.0113 | 4.0882 | 2.99829 | 3.1656 | 0.3694 | 0.7699 | |
| 10 | 4.0116 | 4.1080 | 2.9836 | 3.198 | 0.3822 | 0.8764 | |
| 12 | 4.0213 | 4.1325 | 2.98437 | 3.2251 | 0.4498 | 1.0248 | |
| 14 | 4.0247 | 4.1337 | 2.9631 | 3.1963 | 0.4718 | 1.0291 | |
Table 2: Values of molar cohesive energy, Gibb’s free energy and Δπi of aqueous sodium dodecyl sulphate combined with sodium chloride and sodium sulphate at different temperatures.
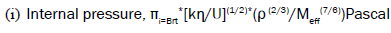 (1)
(1) 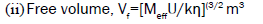 (2)
(2)  (3)
(3) 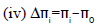 (4)
(4)  (5)
(5) 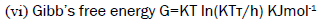 (6)
(6)
Where, U=ultrasonic velocity (m/s), ρ=density (Kg/m3), η=viscosity (Nsm-2), Meff=effective molecular weight, b=packing factor, R=gas constant (8.314*107),
T=temperature (Kelvin), k=temperature independent constant (4.28*109), K=Boltzmann’s constant (1.3806*10-23).
Internal pressure
The measurement of internal pressure is important in the study of the thermodynamic properties of liquids. The internal pressure is the cohesive force, which is a resultant of forces of attraction and forces of repulsion between the molecules [3,4]. The internal pressure increases with the increase in concentration, and decreases with respect to temperature. This decrease in internal pressure indicates the decrease in cohesive forces and the decrease in forces of attraction. Internal pressure seems to be conditioned by attractive forces whereas free volume is sensitive to repulsive forces. The variation of internal pressure with concentration for two fillers at different temperatures is depicted in Figures 1 and 2.
Free volume
The molecules of liquid are not closely packed and as such there is always some free space between them. This free space is known as free volume. It is a significant factor in explaining the free space and its dependent properties have close connection with molecular structure [5] and it may show features about interactions like ion-solvent, dipole-dipole, solute-solvent interactions. When the solute is added to solvent, the structure of solvent is broken. The available space of solvent in the solution is reduced hence the solution becomes more compressed. So the free volume decreases with raise in concentration. When the temperature rises, the repulsive force between the solute and solvent is more and the free space availability is also increases. So free volume increase with increasing temperature. Figures 3 and 4 shows the change in free volume with increasing concentration of NaCl and Na2SO4. The increase in free volume and the decrease in internal pressure indicate that there exists a strong solute-solvent interaction. Hence the addition of fillers to the surfactant increases the efficiency of SDS.
Temperature dependence of πi and Vf
C.V. Suryanarayana and J. Kuppusamy [6] found that at a given temperature a general equation of the form πs=πi+Am2+Bm where, πi is the internal pressure of solvent, πs is the internal pressure of solution, m is the concentration, A and B are temperature dependent constants, holds good in all electrolytes. A similar relation Vf=Vf0+Cm2+Dm holds good for free volume where Vf0 is the free volume of solvent. Similarly C and D are dependent temperatures. The above equations found to be true in many cases of electrolytes, the same was observed in SDS added with fillers NaCl and Na2SO4.
The values of constants A, B, C and D are computed from the internal pressure and free volume at different temperatures is given in Table 3. The temperature dependence of A, B, C and D for the filler solutions are studied and shown in the Figures 5-8. From the tabulation it is clear that the variation of C and D are opposite to that of A and B.
| Temperature (K) | Internal Pressure * 103 | Free Volume * 103 | ||
|---|---|---|---|---|
| NaCl | ||||
| 298 | 0.88 | -0.02534 | -0.2025 | 0.004895 |
| 308 | 1.752 | -0.00992 | -0.1725 | 0.002255 |
| 318 | -13.298 | 0.2586 | 5.06875 | -0.11269 |
| 328 | -12.725 | 0.1842 | 2.85 | -0.06371 |
| Na2SO4 | ||||
| 298 | 1.3975 | -0.03025 | -0.09625 | 0.003533 |
| 308 | 1.8695 | -0.01497 | -11.081 | 0.1288 |
| 318 | -9.955 | 0.23429 | 1.69345 | -0.07228 |
| 328 | -3.9725 | 0.077955 | 0.66417 | -0.0529 |
Table 3: Values of Coefficients of internal pressure and free volume of aqueous sodium dodecyl sulphate combined with sodium chloride and sodium sulphate at different temperatures.
Δπi
The difference Δπi between πi and πs predicts the nature of solute. Internal pressure is the resultant of the attractive and repulsive forces in the system. Co-efficient A refers to the attractive component and B to the repulsive component. B determines the sign of Δπi. In aqueous SDS-filler solutions ultrasonic velocity is found to increase with concentration. But πi found to decrease with increasing concentration of the solutions. The fall in πi is governed by the above relation and B is found to be negative at low temperatures and becomes positive at high temperatures and hence Δπi is negative at low temperatures indicating that the internal pressure of solvent decreases due to the addition of fillers.
The Δi value is found to be negative at low temperatures and changes its value to positive at higher temperatures. The change in Δπi leads to the conclusion that addition of fillers to SDS changes the nature of SDS to be a structure breaker at the lower temperatures and structure maker at higher temperatures. The Δπi variation is depicted in Figures 9 and 10.
Molar cohesive energy
Molar Cohesive energy is defined as the energy of mutual attractive force of molecules. It is the energy needed for the transition of a molecule from the liquid phase where molecules are very close to each other and interactions are strong, to the gaseous phase where molecules are so far from one another. It is the measure of mutual attractiveness of molecules. It increases with increasing concentration suggests that increasing intermolecular interaction which may be due to the strong dipole-dipole interaction in the system.
Gibb’s free energy
Gibb’s free energy is the energy associated with a chemical reaction that can be used to do work. It is the Thermodynamical potential that measures the maximum amount of non-expandable work obtained from a thermodynamic system at a constant temperature and pressure. This property was defined by Josiah Willard Gibb’s to predict whether a process will occur spontaneously at constant temperature and pressure. Molar cohesive energy and Gibb’s free energy has similar variation as that of free volume and shows exactly reverse trend of internal pressure. This shows that there is appreciable interaction between solute and solvent molecules. Figures 11-14 depict the variation molar cohesive energy and Gibb’s free energy for aqueous SDS added with fillers NaCl and Na2SO4.
Osmotic pressure
Osmotic pressure is the minimum pressure which needs to be applied to a solution to prevent the inward flow of solvent molecules across a semi-permeable membrane. It is also defined as the measure of the tendency of a solution to take in water by osmosis. Osmotic pressure is the basis of filtering ("reverse osmosis"), a process commonly used to purify water. It is applied to water purification and desalination, waste material treatment, and many other chemical and biochemical laboratory and industrial processes.
From the Figures 15 and 16, it is observed that the osmotic pressure of the solution at a given temperature is directly proportional to the concentration of filers. The osmotic pressure of NaCl solution is more compared to Na2SO4 solution at all temperatures and concentrations.
Comparison of NaCl and Na2SO4
In the NaCl structure there exists only a single Na+ ion and Cl- ion, whereas in the case of Na2SO4 structure there are two Na+ ion, four O- ions and a sulphate ion present. Due to this reason, the strength of interaction in filler sodium chloride with solvent aqueous SDS is lesser comparative to filler sodium sulphate. The interactions between the solute particles and solvent molecules are more in sodium sulphate. Hence all the Thermodynamical parameters calculated have greater influence in sodium sulphate and solvent molecules than that of sodium chloride. This leads to the conclusion that sodium sulphate behaves like effective filler than sodium chloride. It works efficiently and removes stains from clothes and articles quickly.