ISSN:2321-6212
ISSN:2321-6212
Filimonov IA*
Institute of Structural Macrokinetics and Materials Science (ISMAN), Russian Academy of Sciences, Chernogolovka, Moscow 142432, Russia.
Received date: 16/06/2016; Accepted date: 23/06/2016; Published date: 30/06/2016
Visit for more related articles at Research & Reviews: Journal of Material Sciences
Recent Experimental and numerical studies on Combustion Synthesis of sulfides (CSS) in combination with the current Electronic Theory of a Solid Body (ETSB) lead us to the conclusion that the CSS chemical reactions are accompanied by electron transitions inside the sulfide valence zone, i.e., by transitions between the energy states of electrons localized in the valence zone. Such a conclusion only can explain the huge difference of energy of sulfur clusters affinity to an electron from the sulfur ionization potential which is available in literature. In addition to the interrelated aspects of CSS and ETSB We have presented also a small review of the existing literature on electrochemical sources of current and voltage (cells, accumulators).This review is devoted to distributed electrochemical systems and to a certain extent supplements some of the earlier reviews written (with the participation of the author) for the sources of electricity based on SHS solely. As the result, it has been made the conclusion about the advantage of the combustion based sources of electric current (pyrotechnic sources of electric current, PSEC) in comparison with the modern accumulators and bio-cells. The conditions of PSEC stable work are discussed. Incidentally we have also considered a few works concerning materials synthesis and nanoparticles. Nanoparticles are extremely popular now. The authors of the articles concerning such exotic objects use often in their publications unreasonable approximations or/and unchecked facts. In our opinion, the readers have to realize, where and what conclusions of similar authors deviate from the reality or from the classical results received earlier. To prove a solvency and a coherence of new results and representations is the business of innovators themselves, not of others or us. We just reveal some of their frequent shortcomings here. The comparison of the SHS systems on the specific electric energies generated during the synthesis has been also carried out. The energies appear to depend strongly on the composition, green density and the sample electric resistance. We have shown that in the SHS semiconductive systems of a loosed density (SLD) the generated specific electric energy is comparably less than that of a fish slope. Therefore, We have suggested new non-system units based on the specific electric energy generated by a fish slope as a useful measure for the SLD generated electric energies. It has appeared that the greatest quantity of specific electric energy is emitted by the loosed mixture of titanium with its nitride. Nevertheless, We have calculated that even the titanium nitride mixtures contain the overwhelming part of their energy in that of a chemical type (as the enthalpy of the green mixture formation). Both in compact and in SLD systems the specific standard enthalpies of formation of green components or products exceed significantly the electric energy released. Any source of electric voltage or electric current based on the combustion synthesis cannot be compared by the efficiency to electrolytic accumulators (their efficiency is close to unit by the definition). Therefore, We have also considered the general principles of association of such sources into batteries. It is well known that exoemission of electrons (EE) is an inevitable condition of any solid body existence in Nature. Nevertheless, there is still no clear understanding of high energetic EE caused by some of SHS processes. We have given the estimate confirming the possibility of high energetic electrons to be emitted during SHS, reveal the most perspective SHS systems in this relation, and simultaneously discuss the problems of chemically stimulated exoemission of electrons (CSEE) existing now in our opinion.
Sources of electric voltage and/or current; SHS based Galvanic cells; Accumulators and bio-cells; Scales of the electric energy release.
The paper presented is a harmonious continuation of reviews reported previously. Therefore it may repeat estimates and conclusions of in some single points (in the points of continuity only) [1,2]. Nevertheless, the main literature we considered and evaluated here has been published after 2005 and a little bit later approximately.
Prehistory
Combustion is the most ancient and one of the most important natural processes providing the human activity. If in Childhood of the World combustion was used in practice as the simplest source of energy (thermal, light and/or mechanical one), under modern conditions the role of combustion as well as the whole life in general becomes qualitatively complicated. The reason is that over the years we have to deal with more and more difficult phenomena, devices and/or processes if we want to evolve by ourselves and to rise our knowledge of the Nature. In the process of humanity development there are new actual technological problems which can be solved by the methods of combustion. Among them: (i) Synthesis of new materials with new, unique and still unknown properties; (ii) Potential use in factory production of the specific and high-technological effects accompanying the modes of Combustion Synthesis (CS), i.e.: Exoelectronic and exoionic beams, radiation. (iii) Creation of new sources of voltage and/or electric current [3] based on CS (iv) Electric investigations of the increased accuracy for reaction kinetics studies [4,5]. Items (i, ii, iv) are more or less clear and don't demand more detailed comments. Item (iii) is provided by the need to create the sources of electricity with different internal resistances and capable to work at high negative temperatures (near -40°C and below). Since childhood a majority of us remember that the flame of a fire or a gas torch starts flashing, hissing and shining by multi-colored fires if somebody has thrown into it a small pinch of fine-graned sand or, even better, - salts. Only at mature age, when we have already studied physics and chemistry at the higher school we can explain the prime cause of these child observations more or less. Nitrates of alkaline metals, in particular sodium, decay with release of oxygen when they are heated up to 380°C and higher. This may explain the hissing. Forming sodium ions lead to a flame yellow colored, while those of potassium generate the violet flames. A mixture of sodium and potassium salts burns with a multicolored flame [4]. Let's consider the observations in more details and for simplicity we just count the time of a free fall of the crystals from the height of about 1.5 meter. Solution of even such a rough and simplified problem gives us the lower estimate of the time of falling of the order of 0.6 s. Actually, it exceeds the characteristic times of all the physical and chemical processes taking place on the surface of a sufficiently small (≈<<1 μm in diameter) crystal (both the time of heating up to a maximum temperature (<≈1000°C) and the reaction time at the surface: ≈1 ms for lonely particles of the micron size and ≈10 μs for the nanometric particles [12], etc). Evidently, such a falling particle is an ideal object well corresponding to the discrete modeling [8] of inflammation and combustion processes (when the time of heat removal exceeds that of the reaction) As the result, the overheated solid particles (crystals) fall into the colder bottom part of a flame and initiate gas-solid reactions as well as exoemission of ions and electrons. The observed effects are very similar to those which occur when falling meteorites penetrate into the atmosphere of Earth. The only thing that distinguishes the crystals from a meteorite is the mechanism of heating up in course of the falling. The crystals heat up first of all due to the heat received by them from the top, hotter part of a flame, while a meteorite is warmed up due to the friction in air at its extremely high speed of entry into the atmosphere. In contrast to a meteorite the speed of a small crystal dropping freely into a fire isn't so high to significantly influence the crystal temperature. Therefore, one can neglect air friction in this case.
New Electric Sources
Dependent on the product internal electric resistance finally obtained in a reacting sample one may create a source of voltage (if the resistance is sufficiently high) or a source of electric current with the help of SHS (if the resistance is sufficiently low). Choosing a green chemical composition of the CS system and predicting the products composition one may effectively control the electric internal resistance of the source and thereby, create new sources of electric voltage and/or current. Below we will consider this question in more details. Rather simple and clear classification of electric sources on the sources of electric voltage and the sources of electric current will be also given. In the academic literature an ideal Source of Voltage (SV) can be characterized by the current-voltage curve corresponding to the infinite internal resistance (see Figure 1, SV dashed line): RInt ∝ ctgγ, i.e., RSV=Rint|γ≅0>>1. While an ideal Source of Current (SC) corresponds to the curve of zero internal resistance: RSV=Rint|γ≅π/2<<1. (See Figure 1, SC dashed line). An Actual Source of Electricity (ASE) has a finite resistance and, consequently, is represented by the thick or thin solid line inclined at the angle α and located between the SC and SV lines. For an ASE one may just express the ratio of the angles: α (π / 2) and 1−α (π / 2), showing to what extent the ASE can be considered as a source of current and as a source of voltage correspondingly. For example, the value of α=45°C detected in the current voltage measurements means that this ASE is the source of current by 50% as well as the source of voltage by 50%.
In accordance to Figure 1 one may determine the fraction of an ideal source of voltage presented in any Actual Electric Source of Electricity (ASE), ε, as the following: ε≡2γ/π.The closer is γ to π/2 in the ε expression, then the source can be considered as an ideal source of voltage to the greater extent (ε→1) and vice versa (as a source of electric current) at ε<<1 . The ASE presented on Figure 1 by the thicker line is considered as a SV to a greater extent than that one presented by the thinner solid line and vice versa as a SC.
Chemical Sources of Electric Current (CSEC)
Today we cannot imagine our life without sources of electricity of any kind. The vast majority of them are galvanic cells and/ or accumulators. Comparative characteristics of the most widespread commercial accumulators are presented in Table 1. It is obvious that development of CSEC goes in the direction of increase in density of the reserved energy as well as of an enhancement in voltage generated per a single CSEC element. One may see that in the conditions of northern climate the best modern galvanic cells can't work. There is no such a rigid restriction for the sources based on CS. New charged Lead-Acid Accumulator (LAA) has the internal resistance of about 10-3 Ω=mΩ. As well as for other CSEC, this value continuously grows with aging and/or discharge. LAA isn't perfect in respect of mechanical loadings and leakage of the acid is thus very possible. LAA is bulky and conservative by its form (a rectangular box, usually). In relation to LAA, the Li containing sources maybe produced in a bigger variety of shapes (from thin plates to traditional boxes or cylinders) and are applicable in molding. The only disadvantage of the cells containing lithium consists in their dangerous potential of fire and explosion connected with the high reactivity of lithium. Thus, use and utilization of lithium cells represents certain difficulties if not to mention their ecological harm.
Bio-cells
see from them that the main disadvantages of the biological sources of electricity (bio-cells) with respect to the artificial CSEC consist of their extremely low energetic density (five orders lower than that at artificial CSECs) and of sufficiently high working temperatures necessary for operation of these living beings (1.5÷30°C). Actually, the amplitude current-voltage characteristics of bio-cells (80÷300 V, 1÷7 A) aren't inferior to the similar characteristics of artificial CSEC and sometimes even surpass them. Death of the famous Australian naturalist and a TV host, Steve Irwin, in September of 2006 from a shot of a fish slope is an additional certificate to this point. Table 1 is made in many respects according to commercial data. Therefore, the non-system units are widely used in it. For physicists and chemists we present some estimates in the System Units (SI). According to these estimates the electric specific (per weight unit) power generated by a fish slope equals up to ≈28÷30 W. Correspondingly, because of a low time of the discharge (≈0.03 s) the electric energy released by the fish is up to 0.84 J/kg or in the units of the specific electric power: up to 1.5 W/kg. It's interesting to note that a human body releases 100÷150 W of the thermal power under normal conditions to keep the body temperature. As one can see, the corresponding specific thermal power of a human (<≈2 W/ kg) is comparably less than the electric specific power of a fish slope. These values determine both the similarity and difference between warm-blooded animals and fishes. Human body is an effective thermal generator. While a fish slope is an effective biogenerator of electricity to the same extent approximately (Table1). For reference, the heat released by the Ti+C mixture equals to≈55,3 kcal/mole [9]. If We take into account the TiC molar mass, We can approximately find the specific energy release of one of the most high-temperature compact SHS systems:
| Type | Lead− Acid | NiCd | Ni−MH | Li−ion | Li−ion polymer | Bio−cells, fish slope |
|---|---|---|---|---|---|---|
| Start year of sales | 1970 | 1950 | 1990 | 1991 | 1999 | Notfor sale |
| Density, W×hr/kg | 30−50 | 45−80 | 60−120 | 110 − 160 | 100 − 130 | ≈0,0002 |
| Time of charging,hrs | 8−16 | 1 | 2−4 | 2−3 | 2−3 | − |
| Discharge, per month,% | 5 | 20 | 30 | 10 | 10 | − |
| Maximum number of charge− discharge cycles | 300 | 1500 | 500 | 1000 | 500 | 2÷10 |
| Voltage of an element, V | 1.25 | 1.25 | 1.25 | 3.6 | 3.6 | 80÷300 |
| Need to discharge, times | 2 times in a half of a year | Once per month | Once for 3 months | 0 | 0 | 0 |
| Minimum Working temperature,°C | −20 | −40 | −20 | −20 | 0 | 1,5 |
Table 1: Comparative characteristics of the most widespread modern accumulators and bio-cells.
| No | Mol | Tad, K | Vf, L | Al (G), mol |
O(G), mol |
Al2O3(G), mol |
AlO (G), mol |
AlO2(G), mol |
Al2O (G), mol |
Al2O2 (G), mol |
O2(G), mol |
Al2O3(L), mol |
Al2 (G) mol |
Al (L) mol |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.1Al+3O2 | 1110 | 275 | - | - | - | - | - | - | - | 2.925 | 0.05(S) | - | - |
| 2 | 0.5Al+3O2 | 2904 | 672 | - | 0.210 | - | - | - | - | - | 2.52 | 0.25 | ||
| 3 | Al+1.5O2 | 3873 | 497 | 0.0365 | 0.889 | 0.0001 | 0.162 | 0.0040 | 0.0225 | 0.0032 | 0.3962 | 0.3732 | ||
| 4 | 2Al+1.5O2 | 3970 | 587 | 0.2182 | 0.7 | 0.00024 | 0.448 | 0.0055 | 0.213 | 0.0138 | 0.1441 | 0.4371 | 0.00006 | 0.00006 |
| 5 | 10Al+O2 | 2413 | 1345 | 0.086 | - | - | - | - | 0.572 | - | - | 0.4761 | 0.0006 | 7.817 |
Table 2: Adiabatic equilibrium temperatures as well as the equilibrium phases in the xAl+yO2 (G) systems, P=const, V0=100L, Vf is the final gas volume.
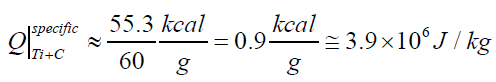
Thus, compact SHS systems can store much more energy than bio-cells and are out of the competition with them energetically
New non-system units reserved chemical energy and released electric energy:
As we have seen from Table 1, non-system units are sometimes useful for certain investigation purposes (like HP for sport cars). For future purpose, we'd like to compare various SHS systems (on composition, conductivity and structure) by their ability to generate electric energy.
In our opinion a new non-system unit expressing the Fish Electric Discharge Energy Specific (FEDES) could be very helpful for such a purpose: 1 FEDES ≡ 0.84 J/kg. For example, the specific energy of electric discharge during oxidation of the Fe/Fe2O3 loosed mixtures [10], χFe/FeO has the following value in FEDESes: χFe/FeO ≅ 0.061 ÷ 0.084 J/kg=0.07÷0.1 FEDESes while the specific energy of electric discharge during oxidation of the Ti/TiO2 loosed mixtures [10], χTi/TiO, equals to χTi/TiO=0.181÷0.2405 FEDESes. Thus, the loosed titanium powders are the more effective generators of electric energy during oxidation than the iron powders. Nevertheless, as well as the iron powders they are also weaker with respect to a fish slope in this regard. Alternatively, there are the SLD much more conductive at normal conditions (n.c.) than iron or titanium oxides (which are actually semiconductors at the n.c.). They appear to be the more effective electric sources even than a fish slope itself. For example, the specific electric energy released during nitridation of the Ti/TiN green mixtures [6], χTi/TiN, exceeds that of a fish slope: χTi/TiN ≅429÷2143 FEDESes and, actually kiloFEDESes (kFEDES) can be suitably applied for them: χTi/TiN ≅429÷2143 FEDESes. 1 k FEDES≡103 FEDES. Using the data of Table 3, we can calculate the ratio between chemical energy reserved initially by SLDs and the electric energy released by them during their combustion synthesis. Therefore, another non-system units which may be also comfortably introduced to estimate the specific electric energy release in this relation, is the specific standard enthalpy of formation, SEF: 1SEFTi/TiN=334 kJ/ mole, and χTi/TiN≅0.11÷0.54 m SEFes, 1 m SEF≡10-3 SEF. As the result, one can conclude that FEDES appears to be a good scale of the electric energy released in semiconductive SLD, while kFEDES or mSEF correspond properly to the magnitude of that in a high conductive SLD (at the n.c.).
| TiN | TiO | Ti2O3 | TiO2 | FeO(S) | FeO(L) | ZnS(S,L) (100K<T<≈1293K) |
|---|---|---|---|---|---|---|
| -334, kJ/mole, |
-526, kJ/mole |
-1518 kJ/mole |
-944kJ/mole (anatase), 939kJ/mole(rutile) | -272 kJ/mole |
-251 kJ/mole |
-0,02÷-61kJ/mole, -201÷203 |
Table 3: Standard Enthalpies of formation of titanium nitride, titanium and iron oxides as well as that of zinc sulfide.
Relation between Specific Electric Energy and Enthalpies of Formation
We have shown above that the specific electric energy emitted during SHS is limited from the top by the values of FEDES or mSEF for the SLD systems. The SEF scale main disadvantage is that it has a different value for various chemical compositions (Table 3). Therefore, FEDES is a more universal unit to compare the specific electric energies released during the combustion of the different chemical compositions while SEF is preferable for comparison of the sources with a similar energetic efficiency (ratio of the released electric energy to the enthalpy of formation). One can see that the similar to SLD but even a sufficiently more rigid restriction is also valid for pressed and compact SHS systems or Systems of Compact Density (SCD) which have usually the electric conductivity (σ) higher than that of SLD systems. The SCD higher electric conductivities as well as the higher thermal diffusion related to their σ values lead to a faster combustion and higher combustion temperatures in them. Unfortunately, the general problem concerning SCD doesn't allow a rather simple measurement of electric currents inside the SCD reacting samples (the surface currents and temperatures are mainly known). Nevertheless, we may use also the upper currents detected in SLD as the corresponding limits for this purpose. Universal Specific standard enthalpies of reagents or products formation (USEF, 1 USEF≡100 kJ/mole) might be chosen instead of FEDESes or SEFes in this case. In contrast to SEF, USEF is a constant and is well comparable with the enthalpies for the majority of systems (Table 3). As one can see, the enthalpies correspond usually up to the values of hundreds kJ/mole (Table 3) and greatly exceed that of the electric energy released (10-6÷10-3 J/mole) in the majority of SCD systems. In the systems with dielectric or semiconductor conductivities (such as ZnS) the detected electric currents correspond to microamperes. Correspondingly, the bottom estimate of specific electric energy release appears to be valid for them: χZnS<≈10-9 USEF. While the upper estimate of the energy in compact SHS systems of relatively high electric conductivity gives the value about three orders higher: χMeN<≈10-6 USEF. In the all other SHS systems the specific electrical energy released appears to be between these two finally estimated limits: ≈10-9 USEF<χSHS<≈10-6 USEF.
Electric SHS Batteries and Their Simplest Classification
Thus, the literature analysis and the Volt-Ampere characteristics of the signals generated by various SHS systems detected lead us to the conclusion that the specific electric energy emitted during SHS is much lower than the specific enthalpy of the green mixture formation. In other words, pure SHS systems alone by themselves are very inefficient in respect of a direct transformation of the reserved chemical energy into the electric one. It would seem that this is a severe and final sentence, and there is no any hope on the SHS as a source of electricity. Nevertheless, the life appears to be richer and much more complicated. By analogy with galvanic batteries and simultaneously with the electric investigation of the combustion synthesis in separate particles or small samples [11-14], the idea of association of separate combustible elements into batteries has been first stated and is now successfully developed in Russia [15-17]. Just as the shock wave is formed by a numerable combination of usual weak waves, the SHS electric battery can be formed by a numerable combination of particles or small samples. It is possible to use the absolutely different ways of association of combustible elements into batteries and/or change the number of elements. Nevertheless, fortunately, the batteries can be easily classified depending solely on a ratio between the battery electric internal resistance, Rint, and the resistance of loading, Rl. The battery is so called a source of electric voltage if the electric current through loading appears to be much less than the current via the battery, i.e. Rint<<Rl. Alternatively the battery serves as a source of electric current if Rint>>Rl. Almost all the current flows through loading in this case. Varenykh NM, et al. [16] has described the battery as a high-temperature reserve source of electric current of one-time action. In contrast to current galvanic cells the batteries provide a higher specific electric power generated in the significantly wider range of working temperatures (-60÷+70°C) [17]. In addition to the temperatures the very important advantage of the SHS batteries concerns to an absence of discharge currents during their storage. Therefore, the SHS batteries can be stored unlimitedly long in practice. The only disadvantage of the batteries relative to galvanic cells consists in a pulse character of the electric field generated by them. There are rather strict requirements to the batteries as to pulse sources of voltage for different applications. In the best of them the pulse time should be less than 0.3 s approximately [17].
LAA History
Lead Acid Accumulator (LAA) is the first type of accumulators developed by a human. There are at least 3 types of LAA. The most widespread type of LAA consists of 6 cells with the voltage of 2.1 V on each of them. Lead, oxide of lead and sulfuric acid are the main three components determining the LAA chemistry. Unfortunately, the pure lead is too soft and the plates from it don't possess the mechanical durability necessary at operation. The softness problem was initially solved by antimony and transition to the lead-antimonial plates as electrodes. It was found later that these plates catalyze hydrolysis of water in the accumulator and result in a need of continuous restoration of water level in it. In practice it looks as boiling of electrolyte and a continuous release of vials of hydrogen and oxygen during the operating time. This results in need of continuous service of the accumulator, replenishment of water level during its operation. The boiling problem was solved by addition of calcium into the electrodes composition. The effect from calcium was so great that at one time producers have once declared creation of a LAA not demanding service. Later it became clear that at the lead-calcinated LAA the quantity of possible charge- discharge cycles sharply decreases. Currently producers have stopped mainly on use of a hybrid LAA combining lead-antimonial and lead-calcinated electrode plates since the plates have minimized the additional required maintenance of LAA on a reasonable and acceptable level for customers.
Li-Ion Chemistry
In course of charging the following reactions occur on the Li-ion accumulator (LiiA) positive plates which
are usually made from Li covered by CoO2: Li CoO2→Li-x CoO2+xLi++xe- (1), while the other reactions take place on the LiiA negative plates (done of carbon usually): C+xLi++xe-→CLix (2), The charging purpose is to create a stock of the spatially divided charges: the positive ions of lithium on a the LiiA positive electrode of and electrons - on the LiiA negative electrode. During the discharge electrons will move to the positive electrode and compensate charge of the ions. A completely discharged accumulator has zero charges both on the positive and negative electrodes, i.e., neutral compounds, LiCoO2 and CLix have been formed on them after the discharge.
It has been noted that the limited storage capacity of lithium ions in LiAs because of the electrodes made of Activated Carbon (AC). Carbon nanotubes, graphene and carbon nanofibers seem to be more suitable materials instead of AC in LiA [18]. Nevertheless, the high cost of these materials prevents the introduction of them into operating LiAs. Another alternative is Silicon and/or silicon- carbon nanocomposites. While pure silicon electrodes reveal a poor mechanical integrity to the LiA operating conditions, the silicon-carbon electrodes appear to be a more suitable substitution to AC in LiAs [19].
New Materials
Researches on creation of new materials are in focus of papers published in the World the last five years. In our opinion, the most promising materials in this direction are now related with so called max- phases [19]. These phases (such as Ti2CdC, Ti2GeC, Zr2InC, Ti3SiC2, Ti4AlN3, and others have been discovered with the help of exfoliation procedure including HF treatment (etching) and subsequent separation of nanosheets by sonification in methanol [19]. These materials combine the properties previously considered as mutually excluding. They maybe for example refractory and, at the same time, maybe easily molded at tool processing. It has been now shown [20] that many of them maybe also produced by a combination of CS and shift deformations. On the other hand, the combination of high energy ball milling and spark plasma sintering (as a CS) provides preparation of Cu- Cr nanocomposite powders with the grain size of 5 nm [21]. Thus, CS plays an important role as a tool for the modern complex technologies of receiving new materials.
CS Working Conditions
The predicted high potential of the CS sources of voltage or electric current under northern climate conditions demands from us an explanation of the precautions which have to be provided for their stable work in practice under these conditions. Surprisingly, but these precautions are rather well-known already today. The matter is that all the precautions follow from the classical theory of limits of thermal flame propagation [22]. The combustion temperature has to correspond to the values from semi-island of the initial mixture inflammation. Decrease in the combustion temperature by more than one characteristic interval will lead to the significant (>e1/2) decrease in the front propagation velocity and subsequent combustion extinction. Therefore, for the CS electric sources it is necessary to choose the SHS compositions with the highest possible temperatures and velocities of the combustion.
History
Despite the fact that the concept of Electrochemical Potential (EP) is known long ago and widely, there is still no finally established understanding of the nature mechanism for emission and radiation effects during CS. Set of current problems promote such a situation. One of the problems is that a system of charges with purely Coulomb interaction between themselves couldn't be in a mechanical balance without some force of unelectric nature keeping away the opposite charges which attract each other [23]. Vice versa, moving charges can be in an equilibrium state and J.J. Thomson [24] has effectively used this fact in the theory of matter (centrifugal force). We had a similar situation in CS. As we know, charge carriers are generated during CS of vast majority of systems. Nevertheless, to explain the EP generated in each of them we should find the reliable mechanism of these carriers separation in each case. Without separation (at least for a time sufficient for the EP detection) the opposite charge carriers have to recombine and produce neutral products only. No electric and/or magnetic field would then be detected during the combustion stage.. C. Wagner was the first modeling and explaining charge generation and transfer in condensed reactive systems [25-27]. He considered the models with the two types of charge carriers only (one positive and another negative) and first predicted diffusion as the mechanism of division of charges. It's clear that rather simple and double systems only can be directly described by Wagner's models. Usually, in practice we deal with more difficult systems when the number of carriers can be even three and more [11,28,29].
Emission Problems
Another problem proceeds from our insufficient knowledge of exoemission: the energy of an emitted electron has to be below some threshold [30]. In accordance to quantum mechanics, the electron can get (tunnel) through a threshold barrier in the case of rather narrow width of the barrier only. Therefore, the exoemission of electrons is mainly provided by those of them which are trapped on shallow energy levels. Deeply trapped electrons are emitted with the help of another exoemission mechanism (for example by dislocations [4]). Unfortunately, modern ideas of an exoemission of deeply trapped electrons are exclusively poor. There is practically nothing else, except for the dislocation mechanism [4]. Nevertheless, experimental data [31] force us to claim that the exoemission of deeply trapped electrons really occurs in course of CS. Maksimov YM, et al. [31] have written about possible emission of electrons with energies up to 150 eV in their experiments .Note, that exit work in polycrystals of single elements ranges from 2.22 eV in potassium up to 5.27 eV in iridium [32]. The characteristics of the majority of metals lie in an interval between these two values. They have described also the distinctive features of the Emission currents accompanying CS [31]. In this way they have reported about the wavy type dependence of the current amplitude as a function of the CS front coordinate. In addition to these low-frequency current oscillations (0.1÷0.3 s-1) they have detected also the high-frequency oscillations (up to 100 MHz) observed during CS only. Nevertheless, all the observations are mainly phenomenological and don't allow everybody to understand more deeply the nature of emission currents peculiar to CS [31]. Actually, question about the CS emission nature has deeper roots closely attached to the question about the nature of chemically stimulated exoemission eclectrons if possible. [30]. Now researchers distinguish some, limited number of types of an electronic exoemission. First of all they write about the thermally and/or optically stimulated exoemission of electrons (TSEE, OSEE). After Mints RI [30] almost nobody acknowledges the possibility of Chemically Stimulated Exoemission of Electrons in a pure form (CSEE). There is no logic in such an incident, since we know a lot of chemical reactions in solids (SHS, etc.) providing rather high temperatures to cause TSEE. Therefore, according to pure logic, CSEE has to exist in nature in any form. Extremely low amplitudes of emission currents are noted [33]: IE ≈10-19 A. Therefore, the attention of researches was concentrated on mechanisms of exoemission intensification. Dislocation Exoemission of Electrons (DEE) is based on the microscopic amplification of emission currents by the electrons deeply trapped by dislocations in monocrystals [4]. DEE provides such an amplification up to IE ≈10-17 A. Unfortunately, the DEE opportunities are limited to a certain charge of dislocations, etc. For more noticeable increase in IE it is necessary to investigate a huge set of chemically reacting compositions. In our opinion, simpler and convenient way in this direction is connected with the macroscopic mechanisms of exoemission intensification, such as convection during CS or the application of an external field (centrifugal [34], gravitational [35,36] and/or electromagnetic, etc. [11,29,36]). It has been also detected a broadband radiation in a distant ultraviolet area of the optical spectrum [37] (upto 200 nanometers, nm) during the CS of heterogeneous systems with solid products. Kirdyashkin AI, et al. [37] have shown that the UV radiation is formed at the combustion in various gases (helium, argon, nitrogen) and has the maximum of intensity in helium at the pressure of 25 kPa. They have explained the radiation by the effects of chemo-ionization, charge separation and subsequent microscopic electrical breakdowns in products. Using resonance interaction of the radiation detected, one may create advance optic materials with a scale of nanostructure not exceeding 200 nm [38]. It has been experimentally proved that high energy electron beams (≈10 keV) may ignite the combustion in an hydrogen-oxygen mixture at the extremely low initial gas pressures (about 500 Pa) as well as lead to the visible and infrared radiation (≈310÷589 nm) [39].
Current State of the Exoemission Problem in SHS
Thus, the CS exoemission of electrons detected recently still needs at least the estimates showing an obvious opportunity or a high probability of CSEE [31]. We are going to present such an estimate in this review. It is well-known that the TSEE electrons have energies, ETSEE, approximately equal to ETSEE ≈ kT (1), where T - is the temperature of an emitting crystal. To estimate energy of the TSEE electrons emitted by CS we just use TC, the combustion temperature instead of T in ratio (1), i.e. for the commonly generated combustion temperatures TC ≅500÷4000 K [40] we obtain: ETSEE ≈6.9 × 10-21 ÷5.52 × 10-20 J=10÷ 100 eV (2), i.e., we got the value of the same order which has been specified in [31]. Therefore, we may fairly believe that the energy of chemically stimulated exoemission electrons, ECSEE, equals to: ECSEE ≅10÷100 eV. As a result, the combustion temperature is higher; the energy of the emitted electrons is greater. Therefore, among a set of SHS-systems the most high-energetic electrons (≈100 eV) will be emitted in systems with the greatest combustion temperatures, i.e. in ZrC and HfC or HfB2 (see [40]). The only question which remains still actual according to estimate (2) consists that the modern theory of dislocation exoemission is developed in relation to monocrystals, and we hardly can apply its results directly to polycrystals commonly used in SHS. Despite the remained question, the estimate we received in (2) confirms conclusions about possible exoemission of high-energetic electrons in course of CS [40].
Exit Work of Electrons
Exit work of an electron from metal or semiconductor is the most important characteristic of the emitter (EW). Without chemical reactions [41] or when they proceed close to a balance, EW as well as the reaction activation energy, Eact, determines the CS reaction mode and the emission characteristics [11]. There are many different ways of the emission intensification. One of them is TSEE, i.e., warming up of the emitting electrode [41]. The others commonly used ways include covering of the emitting electrode by the materials with lower EW (by adsorbates: AgO, Cs, AuO, Au-C, C-Cs, Be-Cs, Ce-Ba, Cr-Cs, where EW≈1÷2 eV).
Dee Peculiarities
DEE, or dislocation exoemission of electrons, develops during plastic deformations and accompanies movement of the dislocations in volume of a monocrystal. The charge of dislocations reaches up to a half of an electron per the lattice knot (in the ZnS monocrystals as the example). This leads to emergence of electric field with the intensity of about EDEE ≈108 V/cm. Such a field accelerates electrons and lets them be emitted into vacuum with the energies of 5÷7 eV. Note, that this value exceeds the estimated intensity of electric breakdown in porous dielectrics almost on three orders of the amplitude and can explain in any degree the optical effects observed in [31,37,42].
Zone Structure of Electron States in Non-reactive Solids
According to the classical electronic theory of a solid body [41], the states of an electron in any solid are characterized spatially by periodic wave functions (the theorem of Bloch, the Bloch functions). Own values of these functions form some alternation of areas (zones) of the energetically resolved and energetically forbidden values. Therefore these zones have been called as the resolved and forbidden zones respectively.
Ionization in Solids
The forbidden zones as well as the resolved zones (the conductivity and valent zones) in dielectric, semiconductor and conductor are presented schematically in Figure 1 [55]. In agreement to this scheme, the process of ionization in a non-reactive solid is currently treated as an electron transition from the valent zone to bottom of the conductivity zone. Therefore, one may estimate the potential of ionization in a non-reactive solid as width of the forbidden zone approximately: PI ≅ΔEg (i) (3). Consequently, since the forbidden zone thickness is the greatest in dielectrics, they have the highest potential of ionization (Figures 2).
Charge Formation in Reactive Solids
Nevertheless, Resent investigation of charge generation and transfer during the CS of sulfides [29,36] lead us to conclusion that vice versa may happen in reactive solids. The reason is that sometimes charge is formed by electron transitions inside the valent zone which has much narrower range of resolved energies. For example, due to sufficiently small energy of sulfur affinity to an electron in comparison with the ionization potential, formation of the negative ions of sulfur is sufficiently more probable than its ionization up to positive ions and actually happens in course of the ZnS synthesis:
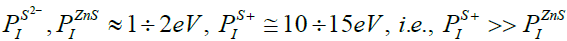
Here we have to notice that electron transitions inside the valent zone are possible only in the case, if this zone is filled not completely, i.e., there are still free levels of energy in it, which can be subsequently occupied by electrons at their redistribution per energy levels during the reaction.
The CS State of Art
Today there is a clear understanding in the combustion community that as a whole at this stage of development the experimental science and experimental results are in advantage to the combustion theory. Researchers often receive experimental results without waiting the corresponding combustion models and without clear understanding of the processes studied. As the example one may consider the discovery of max-phases. There is no still theory and the models explaining the reason of their extremely unique properties. The same concerns the detection of high-energy electrons [31,37]. There is no combustion model considering dislocation development in polycrystals. The existing theories concern just monocrystals at about room temperatures and their applicability to the actual CS is highly questionable. Perhaps, the only thing that kicked out from this row is connected with the complex gas dynamics recently found numerically at research of CCSO and of CSS in open air (numerically and experimentally). Unfortunately, experimental confirmation of gasdynamic calculations [43] is hardly complicated and is now in a development stage. The similar situation happens now in description of nanometric objects (particles, foils etc.). Many successful experimentators and their students have started numerical investigations without a sufficient experience in this field [12]. They have obtained some unreasonable results and try to explain them by the theories non-consistent with the classical combustion [22] and high-temperature oxidation [59]. Have broken the general consistency of the CS science they suggest models contradicting not only to previous investigations[13,14] but even to the common sense. Unfortunately, publication in a top physical Journal isn't a guarantee from mistakes. The top editors very often reject papers concerning narrow specific themes; therefore, they are not able to correctly estimate the newest and contradictive results [12]. As the result, the wrong predictions and views are widely and quickly spread everywhere. The situation is also complicated by the fact, that to check such predictions and views one need extremely expensive installations and the major of peoples prefer just to keep silence.
Combustion Synthesis (CS): Solid or Heterogeneous, Pros and Cons
Due to the fact that the majority of CS systems readsorb a large amount of impurity gas during the combustion [44], a lot of the combustion community members consider CS as a heterogeneous process [45]. We may repeat after the inventor of SHS [46] that CS proceeds in a solid phase. But it wouldn't be absolutely ethic since Merzhanov himself cannot disprove or support us. Therefore we suggest here the estimates emphasizing his correctness and the enviable power of his predictions. To prove such point of view with the arguments we use the experimental results determined by Merzhanov AG, et al. [44]. It has been shown, for the vast majority of the CS systems (from Ti-B, Zr-B, Hf-B, Nb-B to Ti-C, Zr-C, Ta-C.) that the sufficient amount of impurity gases is released during the CS in them [44]. For a more complete and convincing argument, we accompany our remarks and results [44] with some estimates here. Merzhanov AG, et al. [44] have detected the gas release from 1.79 mole of the impurity gas as a whole (in Hf-Si) up to 0.3 (Ti-B), (0.4)(Zr-B), and (0.6) (Hf-B). Under normal conditions (the atmospheric pressure and room temperature) the found amounts of gases will borrow from ≈40 (in Hf-Si) up to ≈7 liters (Hf-B) of the adsorbed gases. Obviously, these values significantly exceed the volume of the condensed products in each case: M(ZrB2)=91+2*11=113 g, ro (ZrB2)=6.09 g/cm3, i.e, v(ZrB2)=688 cm3 << 8960 cm3 of the impurity gas in Zr-B. Since the molar weight of ZrB2 is known:  = 113g as well as the ZrB2 solid density:
= 113g as well as the ZrB2 solid density:  ≅ 6.09 g / cm3 , we can estimate the ZrB2 solid volume as:
≅ 6.09 g / cm3 , we can estimate the ZrB2 solid volume as: 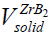 ≅688 cm3 , which appears to be less than the volume of 0.4 mole of gas impurities in ZrB2 [45]:
≅688 cm3 , which appears to be less than the volume of 0.4 mole of gas impurities in ZrB2 [45]: 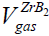 ≅ 8960 cm3 . Under normal conditions,
≅ 8960 cm3 . Under normal conditions, 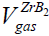 >>
>> 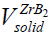 , Nevertheless, at pressure of an inert of ≈200 atm (High Pressure Conditions, or H.P.C.), the volume of the solid doesn't change while that of the gas impurity drops drastically:
, Nevertheless, at pressure of an inert of ≈200 atm (High Pressure Conditions, or H.P.C.), the volume of the solid doesn't change while that of the gas impurity drops drastically: 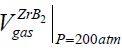 ≅ 45 cm3 and the above gas to solid relation will turn over in the opposite direction:
≅ 45 cm3 and the above gas to solid relation will turn over in the opposite direction: 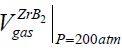 <<
<< 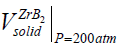 , i.e., as a classic and founder of SHS, A.G. Merzhanov appears probably to be again right in accordance to his definition of a gasless or Solid Flame Combustion (SFC).The exact definition of SFC by Merzhanov says that partial pressures of the all gaseous components should be significantly less than pressure of a surrounding gas [46]. Correspondingly, all the systems adsorbing and releasing rather low (with respect to 1) numbers of moles of impurity gases are combusted in the mode of solid flame propagation [44]. Nevertheless, we should note that such a definition of solid flame propagation is rather formal and not physical one as it isn't based on the mechanism of SHS propagation. There are experiments clearly showing the combustion velocities dependent on partial pressures of adsorbed and impurity gases and proving the heterogeneity as well as a gas content and gas dependence in so-called gasless systems [47,48].
, i.e., as a classic and founder of SHS, A.G. Merzhanov appears probably to be again right in accordance to his definition of a gasless or Solid Flame Combustion (SFC).The exact definition of SFC by Merzhanov says that partial pressures of the all gaseous components should be significantly less than pressure of a surrounding gas [46]. Correspondingly, all the systems adsorbing and releasing rather low (with respect to 1) numbers of moles of impurity gases are combusted in the mode of solid flame propagation [44]. Nevertheless, we should note that such a definition of solid flame propagation is rather formal and not physical one as it isn't based on the mechanism of SHS propagation. There are experiments clearly showing the combustion velocities dependent on partial pressures of adsorbed and impurity gases and proving the heterogeneity as well as a gas content and gas dependence in so-called gasless systems [47,48].
The only one thing that explains the position of Merzhanov and our others colleagues supporting the conception of SFC is related to the fact that often an inert gas of high pressure (100-200 atm.) is used during CS [49-55]. Considering such pressures as well as the low of ideal gases one may see that the volume of a condensed product may be after all also greater than that of the gaseous impurities and one should consider CS as a solid flame in this sense (see Figure 3, H.P.C.) [56]. The TaB2 system is the one only which is actually a heterogeneous system since 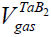 >
> 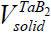 both for N.C., and H.P.C. (Table 4).
both for N.C., and H.P.C. (Table 4).
| CS type | ZrB2 | TaC | Ta-2B |
|---|---|---|---|
| N.C. | 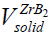 ≅688 cm3, ≅688 cm3,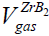 ≅ 8960 cm3 ≅ 8960 cm3 |
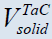 ≅13.4 cm3, ≅13.4 cm3,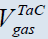 ≅1389 cm3 ≅1389 cm3 |
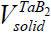 ≅16.4 cm3, ≅16.4 cm3,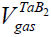 ≅4077 cm3 ≅4077 cm3 |
| H.P.C. | 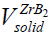 ≅688 cm3, ≅688 cm3,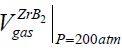 ≅ 45 cm3 ≅ 45 cm3 |
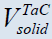 ≅13.4 cm3, ≅13.4 cm3,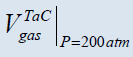 ≅7 cm3 ≅7 cm3 |
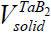 ≅16.4 cm3, ≅16.4 cm3,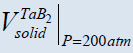 ≅20.4 cm3 ≅20.4 cm3 |
Table 4: Comparison of volumes of impurity gases with that of condensed products at different CS systems.
Nanostructured Combustion Synthesis
In our opinion, the best recent review concerning combustion synthesis of nanostructured systems is written by Rogachev AS and Mukasyan AS [7]. They rejected providently to discuss combustion of nanoparticles in the review frames. We are not going to rewrite here paper [7]. Therefore we just send the reader to this comprehensive material.
Nanoscience
The sharp jump of number of various publications on extremely small-sized (nanometric) systems is now observed. Combustion synthesis is not an exception in this relation. Unfortunately, in a pursuit of newest and unusual results the authors lose often the sense of reality. Therefore we have to pay our attention to some of these exotic examples also. The reason is that nanoscience is now in the early beginning of its formation and it's very important to have the reliable instruments validating safely the most questionable points of any investigations. As we understand philosophy may serve as such an instrument. For example, consider the reactive model of aluminum nanoparticles [12]. The authors use the steady state equation of Poisson equation simplifying the system of temporal diffusion equations [13] used previously and trying to find so called the self-consistent solution which appears to be valid just locally for the equation considered and at the limit of steady-state solution of general system [13]. They forgot the basic principles of philosophy providing the validity of a judgment or a theory. In addition to simplicity, general consistency is the basic and the most important aspect of a valid judgment or theory [57-60]. Quantum mechanics in the quasi-classical limit corresponds to the classical mechanics and at this point is in a consistent interrelation with the last one. Martirosyan KS and Zyskin M [12] have predicted the temperature as well as the generated voltage of aluminum oxidizing nanoparticle independent on the reaction kinetics and surrounding conditions (Figure 1). All the values in their model are determined by the particle mass or size solely. This conclusion is evidently out of the common sense, and breaks the general principle of consistency and contradicts to the experimental data obtained by one of the authors previously [14]. They calculated maximum temperature of the oxidizing particle of as 2000°C. We have checked this conclusion by the thermodynamic calculations. The Al+O2 system temperature varies from about 100 K for the non-stoichiometric compositions) up to 4000 K (for the stoichiometric compositions, Figure 3) It was found previously [16] that reduction of oxygen concentration leads to a weakening of the generated voltage, emergence of pulsations etc. There is no comment on the oxygenation kinetics as well as on oxygen concentrations [12]. Due to the selfconsistent solution found, they consider that oxygen doesn't affect the nanoparticle reactivity, Nevertheless, If this is the case, the drastic existing restrictions applied to a storage(or passivation by inert gases) of nanopowders are completely unclear to everybody today. Actually, there are three well- known philosophical principles of a cognitive science: simplicity, consistency and relevance [61-65]. While the relevance and simplicity indisputable there are solid questions to its general consistency with the other more traditional models, experiments and theories [12]. We have also noted that the great majority of papers concerning nanoparticles or nanofilms are inconsistent globally. Investigating solid phase transitions in the nanometric films (<≈200 nm). Bykova LV, et al. [48] have found that so-called Kournakov temperature (Tk, the temperature of a metal to dielectric transition) governs solid phase transitions in the systems under consideration. On a chemical composition the systems appear to be similar to the SHS ones. Without experience or education in SHS they have, nevertheless, doubted in its consistency and declared a possible key role of the temperature during SHS. Martirosyan KS and Zyskin M [12] and Bykova LV, et al. [48] are our colleagues and even friends. Therefore we have to point out their mistakes certainly. Legendary Socrates told: "Amicus Plato sed magis amica Veritas" (Latin), i.e. Platon is the friend of mine while the truth is closer. We have actually no choice here.
Thus, the analysis of literature available and the results received led us to two rather important conclusions. The first one is that charge generation in reactive solids may be provided by transitions inside of the valent zone. If this is the case, the electric potential of ionization in Reactive Solids (RS) appears to be much lower than that in dielectrics (D): 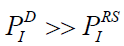 . The second conclusion predict excellent prospect of CS as an electricity source in the conditions of northern climate.
. The second conclusion predict excellent prospect of CS as an electricity source in the conditions of northern climate.
We would like to thank Dr. S.G. Vadchenko for his help in discussions and the thermodynamic calculations ("Thermo", ISMAN).